Advertisements
Advertisements
प्रश्न
Find the volume and the total surface area of a cuboid, whose :
l = 3.5 m, b = 2.6 m and h = 90 cm
उत्तर
Length = 3.5 m Breadth = 2.6 m, Height = 90 cm = `90/100` m = 0.9 m.
Volume of a cuboid = l x b x h = 3.5 x 2.6 x 0.9 = 8.19 m3
Total surface area of a cuboid = 2(l x b + b x h + h x l)
= 2 (3.5 x 2.6 + 2.6 x 0.9 + 0.9 x 3.5) = 2 (910 + 2.34 + 3.15) = 2(14.59)= 29.18 m2
APPEARS IN
संबंधित प्रश्न
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost of white washing the walls of the room and the ceiling at the rate of Rs 7.50 per m2.
The paint in a certain container is sufficient to paint an area equal to 9.375 m2. How many bricks of dimensions 22.5 cm × 10 cm × 7.5 cm can be painted out of this container?
Three equal cubes are placed adjacently in a row. Find the ratio of total surface area of the new cuboid to that of the sum of the surface areas of the three cubes.
An ice-cream brick measures 20 cm by 10 cm by 7 cm. How many such bricks can be stored in deep fridge whose inner dimensions are 100 cm by 50 cm by 42 cm?
A tank is 8 m long, 6 m broad and 2 m high. How much water can it contain?
How many planks each of which is 3 m long, 15 cm broad and 5 cm thick can be prepared from a wooden block 6 m long, 75 cm broad and 45 cm thick?
A village, having a population of 4000, requires 150 litres water per head per day. It has a tank which is 20 m long, 15 m broad and 6 m high. For how many days will the water of this tank last?
A swimming pool is 250 m long and 130 m wide. 3250 cubic metres of water is pumped into it. Find the rise in the level of water.
The dimensions of a cuboid are in the ratio 5 : 3 : 1 and its total surface area is 414 m2. Find the dimensions.
A cloassroom is 11 m long, 8 m wide and 5 m high. Find the sum of the areas of its floor and the four walls (including doors, windows, etc.)
A swimming pool is 20 m long 15 m wide and 3 m deep. Find the cost of repairing the floor and wall at the rate of Rs 25 per square metre.
The perimeter of a floor of a room is 30 m and its height is 3 m. Find the area of four walls of the room.
If V is the volume of a cuboid of dimensions a, b, c and S is its surface area, then prove that \[\frac{1}{V} = \frac{2}{S}\left( \frac{1}{a} + \frac{1}{b} + \frac{1}{c} \right)\]
A closed iron tank 12 m long, 9 m wide and 4 m deep is to be made. Determine the cost of iron sheet used at the rate of Rs 5 per metre sheet, sheet being 2 m wide.
A field is 150 m long and 100 m wide. A plot (outside the field) 50 m long and 30 m wide is dug to a depth of 8 m and the earth taken out from the plot is spread evenly in the field. By how much is the level of field raised?
If each edge of a cuboid of surface area S is doubled, then surface area of the new cuboid is
The sum of the length, breadth and depth of a cuboid is 19 cm and its diagonal is ` 5 sqrt(5)` cm. Its surface area is
The breadth and height of a rectangular solid are 1.20 m and 80 cm respectively. If the volume of the cuboid is 1.92 m3; find its length.
Find the volume and total surface area of a cube whose each edge is:
(i) 8 cm
(ii) 2 m 40 cm.
The total surface area of a cube is 216 cm2. Find its volume.
A wall 9 m long, 6 m high and 20 cm thick, is to be constructed using bricks of dimensions 30 cm, 15 cm, and 10 cm. How many bricks will be required?
Find the volume of wood required to make a closed box of external dimensions 80 cm, 75 cm, and 60 cm, the thickness of walls of the box being 2 cm throughout.
The height of a circular cylinder is 20 cm and the diameter of its base is 14 cm. Find:
(i) the volume
(ii) the total surface area.
375 persons can be accommodated in a room whose dimensions are in the ratio of 6 : 4 : 1. Calculate the area of the four walls of the room if the each person consumes 64m3 of air.
Find the Total Surface Area and the Lateral Surface Area of a cuboid whose dimensions are: length = 20 cm, breadth = 15 cm, height = 8 cm
The areas of any two faces of a cuboid are equal.
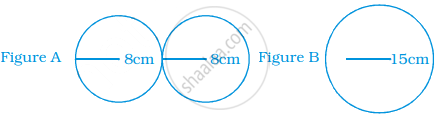
Below are the drawings of cross sections of two different pipes used to fill swimming pools. Figure A is a combination of 2 pipes each having a radius of 8 cm. Figure B is a pipe having a radius of 15 cm. If the force of the flow of water coming out of the pipes is the same in both the cases, which will fill the swimming pool faster?