Advertisements
Advertisements
प्रश्न
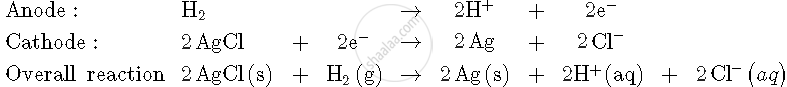
For the reaction
`2AgCl (s) + H_2 (g) ("1 atm") -> 2Ag (s) + 2H^(+) (0.1 M) + 2Cl^(-) (0.1 M)`
`triangleG^0 = -43600 J at 25^@ C`
Calculate the e.m.f. of the cell
`[log 10^(-n) = -n]`
उत्तर
`triangleG^0 = -nFE_(cell)^0`
`:. E_(cell)^0 = 0.226 V`
`E_(cell) = 0.226 - (0.059)/2 log (0.1)^2 (0.1)^2`
Therefore, `E_(cell) = 0.226 - (0.059)/2 log 10^(-4)`
= 0.226 + 0.059 x 2
= 0.344 V
APPEARS IN
संबंधित प्रश्न
With the help of the equation ΔG° = - nFEocell. Explain that cell potential is an intensive property.
Calculate ΔrG° for the reaction
Mg (s) + Cu2+ (aq) → Mg2+ (aq) + Cu (s)
Given : E°cell = + 2.71 V, 1 F = 96500 C mol–1
Potential of saturated calomel electrode is-
What is the effect of catalyst on: Gibbs energy (∆G)
What is the ‘Ellingham diagram’? Write any ‘two points’ of its significance.
The electrical work done in a galvanic cell is equal to ______
The Gibb's energy change (in J) for the given reaction at [Cu2+] = [Sn2+] = 1 M and 298 K is ______.
\[\ce{Cu(s) + Sn^{2+}(aq) -> Cu^{2+}(aq) + Sn(s)}\]
`["E"_("Sn"^(2+)//"Sn")^0 = -0.16 "V", "E"_("Cu"^(2+)//"Cu")^0 = 0.34 "V", "Take F" = 96500 "C mol"^-1]`
The equilibrium constant at 25°C for the given cell is:
Zn | Zn2+ (1M) | | Ag+ (1M) |
Ag is ______ × 1052.
Given that
\[\ce{E^0_{Zn/Zn^{2+}}}\] = 0.76 V
and \[\ce{E^0_{Ag/Ag^{+}}}\] = −0.80 V
The standard Gibbs energy for the given cell reaction in kJ mol-1 at 2'98 K is:
\[\ce{Zn(s) + Cu^{2+} (aq) -> Zn^{2+} (aq) + Cu(s)}\]
E° = 2 V at 298 K
(Faraday's constant, F = 96000 C mol-1)
For a spontaneous reaction, the ΔG, equilibrium constant (K) and `"E"_"cell"^circ` will be respectively.
