Advertisements
Advertisements
प्रश्न
For the `beta^+` (positron) emission from a nucleus, there is another competing process known as electron capture (electron from an inner orbit, say, the K−shell, is captured by the nucleus and a neutrino is emitted).
\[\ce{e+ + ^A_Z X -> ^A_{Z - 1}Y + \text{v}}\]
Show that if `beta^+` emission is energetically allowed, electron capture is necessarily allowed but not vice−versa.
उत्तर
Let the amount of energy released during the electron capture process be Q1. The nuclear reaction can be written as:
\[\ce{e+ + ^A_Z X -> ^A_{Z - 1}Y + \text{v} + Q_1}\] .....(1)
Let the amount of energy released during the positron capture process be Q2. The nuclear reaction can be written as:
\[\ce{e+ + ^A_Z X -> ^A_{Z - 1}Y + \text{v} + Q_1}\] ...(2)
`"m"_"N"(""_"Z"^"A" "X")` = Nuclear mass of `""_"Z"^"A" "X"`
`"m"_"N"(""_("Z"-1)^"A" "Y")` = Nuclear mass of `""_("Z"-1)^"A" "Y"`
`"m"(""_"Z"^"A" "X")` = Atomic mass of `""_"Z"^"A" "X"`
`"m"(""_("Z" - 1)^"A" "X")` = Atomic mass of `""_("Z" -1)^"A" "X"`
me = Mass of an electron
c = Speed of light
Q-value of the electron capture reaction is given as:
`"Q"_1 = ["m"_"N" (""_"Z"^"A" "X") + "m"_"e" - "m"_"N"(""_("Z"-1)^"A" "Y"))]"c"^2`
`= ["m"(""_"Z"^"A" "X") - "Zm"_"e" + "m"_"e" - "m"(""_("Z"-1)^"A" "Y") + ("Z" - 1)"m"_"e"]"c"^2`
`= ["m"(""_"Z"^"A" "X") - "m"(""_("z" - 1)^"A" "Y")]"c"^2` ....(3)
Q-value of the positron capture reaction is given as:
`"Q"_2 = ["m"_"N" (""_"Z"^"A" "X") - "m"_"N"(""_("z"-1)^"A" "Y") - "m"_"e"]"c"^2`
`= ["m"_"N"(""_"Z"^"A" "X") - "m"_"N" (""_("z"-1)^"A" "Y") + ("Z" - 1)"m"_"e" - "m"_"e"]"c"^2`
`= ["m"(""_"Z"^"A" "X") - "m"(""_("z" - 1)^"A" "Y") - 2"m"_"e"]"c"^2` ...(4)
It can be inferred that if Q2 > 0, then Q1 > 0; Also, if Q1> 0, it does not necessarily mean that Q2 > 0.
In other words, this means that if `beta^+` emission is energetically allowed, then the electron capture process is necessarily allowed, but not vice-versa. This is because the Q-value must be positive for an energetically allowed nuclear reaction.
APPEARS IN
संबंधित प्रश्न
Consider the D−T reaction (deuterium−tritium fusion)
\[\ce{^2_1H + ^3_1H -> ^4_2He + n}\]
Calculate the energy released in MeV in this reaction from the data:
`"m"(""_1^2"H")`= 2.014102 u
`"m"(""_1^3"H")`= 3.016049 u
Consider the D−T reaction (deuterium−tritium fusion)
\[\ce{^2_1H + ^3_1H -> ^4_2He}\]
Consider the radius of both deuterium and tritium to be approximately 2.0 fm. What is the kinetic energy needed to overcome the coulomb repulsion between the two nuclei? To what temperature must the gas be heated to initiate the reaction? (Hint: Kinetic energy required for one fusion event =average thermal kinetic energy available with the interacting particles = 2(3kT/2); k = Boltzman’s constant, T = absolute temperature.)
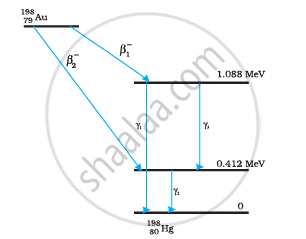
Obtain the maximum kinetic energy of β-particles, and the radiation frequencies of γdecays in the decay scheme shown in Fig. 13.6. You are given that
m (198Au) = 197.968233 u
m (198Hg) =197.966760 u
Write the β-decay of tritium in symbolic form.
Write the basic nuclear process of neutron undergoing β-decay.
Why is the detection of neutrinos found very difficult?
What is the difference between cathode rays and beta rays? When the two are travelling in space, can you make out which is the cathode ray and which is the beta ray?
In beta decay, an electron (or a positron) is emitted by a nucleus. Does the remaining atom get oppositely charged?
During a negative beta decay,
Ten grams of 57Co kept in an open container beta-decays with a half-life of 270 days. The weight of the material inside the container after 540 days will be very nearly
Consider a sample of a pure beta-active material.
Complete the following decay schemes.
(a) `"" _88^226Ra → alpha+`
(b) `""_8^19O → _9^19F+`
(c) `""_13^25Al → ""_12^25Mg+`
A free neutron decays into ______.
