Advertisements
Advertisements
प्रश्न
How many 3-digit even numbers can be formed from the digits 1, 2, 3, 4, 5, 6 if the digits can be repeated?
उत्तर
There will be as many ways as there are ways of filling 3 vacant places 
in succession with the given six digits. In this case, the units place can be filled by 2 or 4 or 6 only, i.e., the units place can be filled in 3 ways. The tens place can be filled by any of the 6 digits in 6 different ways and the hundreds place can be filled by any of the 6 digits in 6 different ways, as the digits can be repeated.
Therefore, by the multiplication principle, the required number of three digit even numbers is 3 × 6 × 6 = 108.
APPEARS IN
संबंधित प्रश्न
How many 3-digit numbers can be formed from the digits 1, 2, 3, 4 and 5 assuming that repetition of the digits is allowed?
A coin is tossed 3 times and the outcomes are recorded. How many possible outcomes are there?
Given 5 flags of different colours, how many different signals can be generated if each signal requires the use of 2 flags, one below the other?
How many two letter words can be formed using letters from the word SPACE, when repetition of letters is allowed?
How many three-digit numbers can be formed using the digits 2, 3, 4, 5, 6 if digits can be repeated?
How many numbers between 100 and 1000 have 4 in the units place?
How many four digit numbers will not exceed 7432 if they are formed using the digits 2, 3, 4, 7 without repetition?
How many five-digit numbers formed using the digit 0, 1, 2, 3, 4, 5 are divisible by 5 if digits are not repeated?
A person went to a restaurant for dinner. In the menu card, the person saw 10 Indian and 7 Chinese food items. In how many ways the person can select either an Indian or a Chinese food?
Given four flags of different colours, how many different signals can be generated if each signal requires the use of three flags, one below the other?
Four children are running a race:
In how many different ways could they finish the race?
How many three-digit numbers are there with 3 in the unit place?
without repetition
How many three-digit odd numbers can be formed by using the digits 0, 1, 2, 3, 4, 5? if the repetition of digits is allowed
Count the numbers between 999 and 10000 subject to the condition that there are no restriction
Count the numbers between 999 and 10000 subject to the condition that there are no digit is repeated
Count the numbers between 999 and 10000 subject to the condition that there are at least one of the digits is repeated
How many numbers are there between 1 and 1000 (both inclusive) which are divisible neither by 2 nor by 5?
Find the value of `(("n" + 3)!)/(("n" + 1)!)`
Evaluate `("n"!)/("r"!("n" - "r")!)` when n = 10, r = 3
Evaluate `("n"!)/("r"!("n" - "r")!)` when for any n with r = 2
Choose the correct alternative:
The sum of the digits at the 10th place of all numbers formed with the help of 2, 4, 5, 7 taken all at a time is
Choose the correct alternative:
In an examination there are three multiple choice questions and each question has 5 choices. Number of ways in which a student can fail to get all answer correct i
Choose the correct alternative:
The number of 5 digit numbers all digits of which are odd i
Choose the correct alternative:
The number of 10 digit number that can be written by using the digits 2 and 3 is
In how many ways can this diagram be coloured subject to the following two conditions?
(i) Each of the smaller triangle is to be painted with one of three colours: red, blue or green.
(ii) No two adjacent regions have the same colour.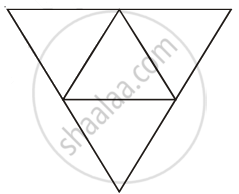
Out of 18 points in a plane, no three are in the same line except five points which are collinear. Find the number of lines that can be formed joining the point
Find the number of positive integers greater than 6000 and less than 7000 which are divisible by 5, provided that no digit is to be repeated.
Find the number of integers greater than 7000 that can be formed with the digits 3, 5, 7, 8 and 9 where no digits are repeated.
The number of different four-digit numbers that can be formed with the digits 2, 3, 4, 7 and using each digit only once is ______.
The sum of the digits in unit place of all the numbers formed with the help of 3, 4, 5 and 6 taken all at a time is ______.
Three letters can be posted in five letterboxes in 35 ways.
In a steamer there are stalls for 12 animals, and there are horses, cows and calves (not less than 12 each) ready to be shipped. They can be loaded in 312 ways.
If the number of five-digit numbers with distinct digits and 2 at the 10th place is 336 k, then k is equal to ______.
