Advertisements
Advertisements
प्रश्न
If a, b, c, d are in continued proportion, prove that:
(a2 + b2 + c2) (b2 + c2 + d2) = (ab + bc + cd)2.
उत्तर
L.H.S.
= (d2k6 + d2k4 + d2k2)(d2k4 + d2k2 + d2)
= d2k2 (k4 + k2 + 1)d2 (k4 + k2 + 1)
= d4k2 (k4 + k2 + 1)2
R.H.S.
= (ab + bc + cd)2
= (dk3·dk2 + dk2·dk + dk·d)2
= d4·k2 (k4 + k2 + 1)2
L.H.S. = R.H.S.
Hence proved.
APPEARS IN
संबंधित प्रश्न
If three quantities are in continued proportion; show that the ratio of the first to the third is the duplicate ratio of the first to the second.
Find the third proportional to a2 – b2 and a + b.
If x and y be unequal and x : y is the duplicate ratio of x + z and y + z, prove that z is mean proportional between x and y.
What number must be added to each of the numbers 6, 15, 20 and 43 to make them proportional?
What quantity must be added to each term of the ratio a + b: a - b to make it equal to (a + b)2 : (a - b)2 ?
Find the mean proportion of: (a – b) and (a³ – a²b), a> b
Write (T) for true and (F) for false in case of the following:
45 km : 60 km : : 12 h : 15 h
If `a/c = c/d = c/f` prove that : `(a^2)/(b^2) + (c^2)/(d^2) + (e^2)/(f^2) = "ac"/"bd" + "ce"/"df" + "ae"/"df"`
If ax = by = cz; prove that `x^2/"yz" + y^2/"zx" + z^2/"xy" = "bc"/a^2 + "ca"/b^2 + "ab"/c^2`
Sleeping time of a python in a 24 hour clock is represented by the shaded portion in figure.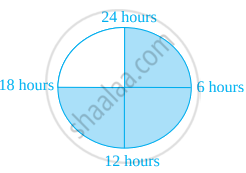
The ratio of sleeping time to awaking time is ______.
