Advertisements
Advertisements
प्रश्न
If r2 =pq, show that p : q is the duplicate ratio of (p + r) : (q + r).
उत्तर
Given, r2 = pq
Duplicate ratio of ( p + r) : ( q + r) = (p + r)2 : (q + r)2
= (p2 + r2 + 2pr) : (q2 + r2 + 2qr)
= (p2 + pq + 2pr) : (q2 + pq + 2qr)
= p(p + q + 2r) : q(q + p + 2r)
= p : q
Thus, p : q is the duplicate ratio of ( p + r) : (q + r)
APPEARS IN
संबंधित प्रश्न
Find the ratio of the following in the simplest fcrm:
432 and 120
Which of the following ratio is greater ?
`5/2 : 15/4` and `5/3 : 11/6`
Given `(x^3 + 12x)/(6x^2 + 8) = (y^3 + 27y)/(9y^2 + 27)`. Using componendo and dividendo, find x : y.
If x : y = 2 : 3, find the value of (3x + 2y) : (2x + 5y).
If a: b with a ≠ b is the duplicate ratio of a + c: b + c, show that c2 = ab.
If a : b = 5 : 3, show that (5a + 8b) : (6a – 7b) = 49 : 9.
Divide 72 in the ratio `2 1/2:1 1/2`
Which ratio is smaller:
`9/17` or `8/15`
The ratio of income to savings of a family is 11 : 2. Find the expenditure if the savings is Rs 1520.
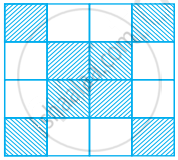
In figure, find the ratio of the area of the shaded portion to that of the whole figure: