Advertisements
Advertisements
प्रश्न
Illustrate-combination of cells e.g., three cells, in parallel, explaining the combination briefly. Obtain an expression for current ‘i’ in the combination.
उत्तर
Combination of cells in parallel: Let there be n cells, each of e.m.f. E and internal resistance r, be connected in parallel as shown in the diagram.
Total e.m.f. across the point A and B is E
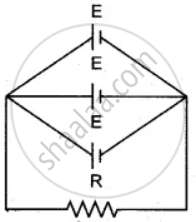
Total internal resistance
`1/"r"_1 = 1/"r" + 1/"r" + 1/"r".... = "n"/"r"` (if there are n cells)
or r1 = `"r"/"n"`
But total resistance of circuit = R + `"r"/"n" = ("nR" + "r")/"n"`
∴ Current i = `"Total e.m.f"/"Total resistance"`
`= "E"/("R" + "r"/"n")`
`= "nE"/("nR + "r"")`
It is advantageous to join cells in parallel when the internal resistance of each cell is quite high as compared to the external resistance R.
Then, Approximately, i = `"nE"/"r"` (neglecting R).
APPEARS IN
संबंधित प्रश्न
If the equivalent resistance is to be increased, then the number of resistances should be connected in
- Series
- Parallel
- Mixed arrangement
- None of the above
Find the equivalent resistance between points A and B.
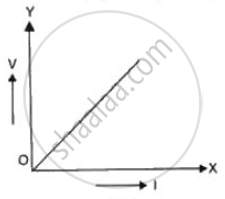
A graph is plotted taking p.d. along y-axis and electric current along x-axis. Name the physical quantity that represents the slope of this graph (Fig.).
Three resistors of 6.0 Ω, 2.0 Ω and 4.0 Ω are joined to an ammeter A and a cell of emf 6.0 V as shown in the following figure. Calculate :
- the effective resistance of the circuit.
- the reading of ammeter.

What is a resistance? Define it with respect to Ohm’s law,
State and explain the laws of resistance.
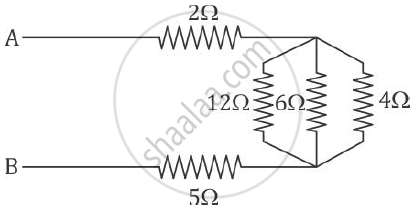
Six resistances are connected together as shown in the figure. Calculate the equivalent resistance between points A and B.

Two wires of same material and same lengths have radii in the ratio of 2 : 3, Compare their resistances.
Two wires of the same length and area made of two materials of resistivity ρ1 and ρ2 are connected in series to a source of potential V. The equivalent resistivity for the same area is:
Two electric bulbs have resistances in the ratio 1 : 2. If they are joined in series, the energy consumed in them is in the ratio:
