Advertisements
Advertisements
प्रश्न
In a parallelogram ABCD ∠C = 98°. Find ∠A and ∠B.
उत्तर
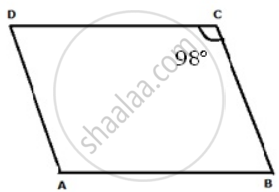
ABCD is a parallelogram
∠C = 98°
∴ ∠A = ∠C = 98° ....(opposite angles of a parallelogram are equal)
∠A + ∠B +∠C + ∠D = 360° ....(Sum of all angles of a quadrilateral = 360°)
98° + ∠B + 98° + ∠D = 360°
∠B + 196 + ∠D = 360°
∠B + ∠D = 360° - 196°
∠B +∠D = 164°
But ∠B = ∠D ....(opposite angles of a parallelogram are equal)
⇒ 2∠B = 164°
⇒ ∠B = 82° = ∠D
Therefore,
∠B = 82°, ∠A = 98°.
APPEARS IN
संबंधित प्रश्न
In the following figure, ABCD and PQRS are two parallelograms such that ∠D = 120° and ∠Q = 70°.
Find the value of x.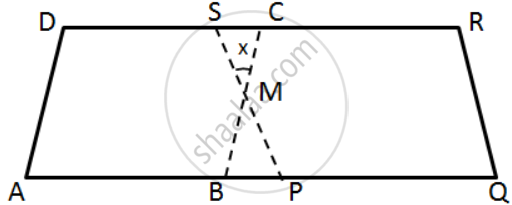
In the given figure, AP is the bisector of ∠A and CQ is the bisector of ∠C of parallelogram ABCD. 
Prove that APCQ is a parallelogram.
In the following figure, ABCD is a parallelogram. 
Prove that:
(i) AP bisects angle A.
(ii) BP bisects angle B
(iii) ∠DAP + ∠BCP = ∠APB
In the following figures, find the remaining angles of the parallelogram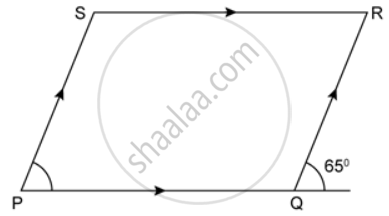
In the following figures, find the remaining angles of the parallelogram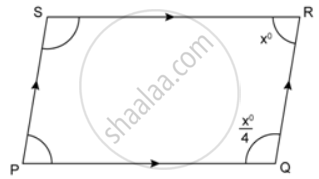
The consecutive angles of a parallelogram are in the ratio 3:6. Calculate the measures of all the angles of the parallelogram.
Find the measures of all the angles of the parallelogram shown in the figure: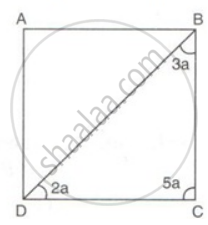
The angles of a triangle formed by 2 adjacent sides and a diagonal of a parallelogram are in the ratio 1 : 5 : 3. Calculate the measures of all the angles of the parallelogram.
Opposite angles of a quadrilateral ABCD are equal. If AB = 4 cm, determine CD.
If PQRS is a parallelogram, then ∠P – ∠R is equal to ______.
