Advertisements
Advertisements
प्रश्न
In fig., circles C(O, r) and C(O’, r/2) touch internally at a point A and AB is a chord of the circle C (O, r) intersecting C(O’, r/2) at C, Prove that AC = CB.
योग
उत्तर
Join OA, OC and OB. Clearly, ∠OCA is the angle in a semi-circle.
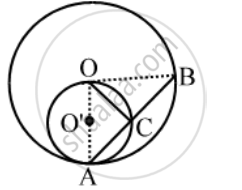
∴ ∠OCA = 90°
In right triangles OCA and OCB, we have
OA = OB = r
∠OCA = ∠OCB = 90° and OC = OC
So, by RHS criterion of congruence, we get
∆OCA ≅ ∆OCB
⇒ AC = CB
shaalaa.com
क्या इस प्रश्न या उत्तर में कोई त्रुटि है?
