Advertisements
Advertisements
प्रश्न
In the given figure, if A(P-ABC) = 154 cm2 radius of the circle is 14 cm, find
(1) `∠APC`
(2) l ( arc ABC) .

उत्तर
The radius of the circle, r = 14 cm
(1)
A(P-ABC) = Area of the sector PABC = 154 cm2
\[\therefore \frac{\theta}{360° } \times \pi r^2 = 154\]
\[ \Rightarrow \frac{\angle APC}{360° } \times \frac{22}{7} \times \left( 14 \right)^2 = 154\]
\[ \Rightarrow \angle APC = \frac{154 \times 7 \times 360° }{22 \times 196} = 90° \]
Thus, the measure of `∠`APC is 90º.
(2) l (arc ABC) = Length of the arc ABC
Thus, the length of arc ABC is 22 cm.
APPEARS IN
संबंधित प्रश्न
How many times a wheel of radius 28 cm must rotate to go 352 m? (Take `pi = 22/7`)
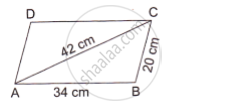
The adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm, and the diagonal AC measures 42 cm. Find the area of the parallelogram.
Find the area of trapezium whose parallel sides are 11 m and 25 m long, and the nonparallel sides are 15 m and 13 m long.
The shape of the cross section of a canal is a trapezium. If the canal is 10 m wide at the top, 6 m wide at the bottom and the area of its cross section is `640m^2` , find the depth of the canal.
The radius of a sector of a circle is 7 cm. If the measure of the arc of the sector is - three right angles; find the area of the sector in case.
Find the radius of a circle whose perimeter and area are numerically equal.
The perimeter of a circular field is 242 m. The area of the field is
The areas of two circles are in the ratio 9 : 4. The ratio of their circumferences is
The radius of the circle is given below; find the area.
28 cm
Find the area of the circle if its circumfence is 88 cm.
A circus tent is cylindrical to a height of 5 m and conical above it. If its diameter is 42 m and slant height of the cone is 53 m, calculate the total area of the canvas required.
Find the area of a circular field that has a circumference of 396 m.
Find the volume and the surface area of the spheres in the following :
Radius = 7 cm
A cylindrical bucket, whose base radius is 20 cm, is filled with water to a height of 25 cm. A heavy iron spherical ball of radius 10 cm is dropped to submerge completely in water in the bucket. Find the increase in the level of water.
A solid sphere metal is cut through its centre into 2 equal parts. If the diameter of the sphere is 3`1/3` cm , find the total surface of each part, correct to two decimal places.
The perimeter of a square and the circumference of a circle are equal. If the length of each side of the square is 22 cm, find:
(i) perimeter of the square.
(ii) circumference of the circle.
(iii) radius of the circle.
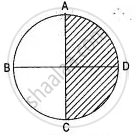
AC and BD are two perpendicular diameter of a circle ABCD. Given that the area of shaded portion is 308 cm2 calculate:
(i) The length of AC and
(ii) The circumference of circle
Construct an angle PQR = 45°. Mark a point S on QR such that QS = 4.5 cm. Construct a circle to touch PQ at Q and also to pass through S.
The cost of fencing a circular field at the rate of Rs.250 per metre is Rs.55000. The field is to be ploughing at the rate of Rs.15 per m2. Find the cost of ploughing the field.
Diameters of different circles are given below. Find their circumference (Take π = `22/7`)
d = 70 cm
