Advertisements
Advertisements
प्रश्न
In how many lines two distinct planes can intersect?
उत्तर
If we look at two intersecting plane, we can see that there is only one unique line at which the two planes intersect.

Therefore, two distinct planes can intersect each other at a single unique line as only a single line is common between two intersecting planes.
APPEARS IN
संबंधित प्रश्न
The following statement is true or false? Give reason for your answer.
Only one line can pass through a single point.
Give a definition of the following term. Are there other terms that need to be defined first? What are they, and how might you define them?
radius of a circle
How many lines can be drawn through a given point.
The number of interwoven isosceles triangles in Sriyantra (in the Atharvaveda) is ______.
If a quantity B is a part of another quantity A, then A can be written as the sum of B and some third quantity C.
Solve the following question using appropriate Euclid’s axiom:
Two salesmen make equal sales during the month of August. In September, each salesman doubles his sale of the month of August. Compare their sales in September.
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have X and Y are the mid-points of AC and BC and AX = CY. Show that AC = BC.
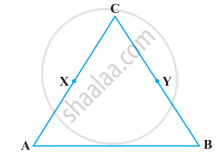
Solve the following question using appropriate Euclid’s axiom:
In the following figure, if OX = `1/2` XY, PX = `1/2` XZ and OX = PX, show that XY = XZ.
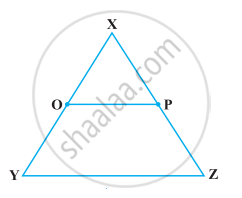
Read the following statements which are taken as axioms:
- If a transversal intersects two parallel lines, then corresponding angles are not necessarily equal.
- If a transversal intersect two parallel lines, then alternate interior angles are equal.
Is this system of axioms consistent? Justify your answer.
Read the following two statements which are taken as axioms:
- If two lines intersect each other, then the vertically opposite angles are not equal.
- If a ray stands on a line, then the sum of two adjacent angles so formed is equal to 180°.
Is this system of axioms consistent? Justify your answer.
