Advertisements
Advertisements
प्रश्न
In the Auger process an atom makes a transition to a lower state without emitting a photon. The excess energy is transferred to an outer electron which may be ejected by the atom. (This is called an Auger electron). Assuming the nucleus to be massive, calculate the kinetic energy of an n = 4 Auger electron emitted by Chromium by absorbing the energy from a n = 2 to n = 1 transition.
Advertisements
उत्तर
Auger Effect: The Auger effect is a process by which electrons with characteristic energies are ejected from atoms in response to a downward transition by another electron in the atom. In Auger spectroscopy, the vacancy is produced by bombardment with high-energy electrons, but the Auger effect can occur if the vacancy is produced by other interactions. It is observed as one of the methods of electron rearrangement after electron capture into the nucleus.
If an inner shell electron is removed from an atom, an electron from a higher level will quickly make the transition downward to fill the vacancy. Sometimes this transition will be accompanied by an emitted photon whose quantum energy matches the energy gap between the upper and lower level. Since for heavy atoms this quantum energy will be in the x-ray region, it is commonly called x.-ray fluorescence. This emission process for lighter atoms and outer electrons gives rise to line spectra.
In other cases, the energy released by the downward transition is given to one of the outer electrons instead of to a photon, and this electron is then ejected from the atom with an energy equal to. the energy lost by the electron which made the downward transition minus the binding energy of the electron that is ejected from the atom. Though more involved in interpretation than optical spectra, the analysis of the energy spectrum of these emitted electrons does give information about dying atomic energy levels. The Auger effect bears some resemblance to the internal conversion of the nucleus, which also ejects an electron.
Sometimes an upper election drops to fill the vacancy, emitting a photon.
As the nucleus is massive, the recoil momentum of the atom may be neglected and the entire energy of the transition may be considered transferred to the Auger electron. As there is a single valence electron in Cr, the energy states may be thought of as given by the Bohr model.
The energy En of the nth state
`E_n = + Z^2 R[1/n_1^2 - 1/n_2^2]`
= `Z^2 R [1/1 - 1/4]` .....[For n1 = 1, n2 = 2]
Z = 24
R = Rydberg constant
∴ `E_n = 3/4 Z^2 R`
The energy required to eject an electron from n = 4 state is `E_4 = Z^2 R 1/4^2 = 1/16 Z^2 R`
The energy given to electron is converted into KE. of the ejected electron.
Hence, the KE. of Auger (ejected) electron = En – E4
K.E. = `Z^2R 3/4 - 1/16 Z^2R`
= `11/16 Z^2R`
= `11/16 xx 24 xx 24 xx 13.6` eV
K.E. = 11 × 36 × 13.6 = 5385.6 eV
APPEARS IN
संबंधित प्रश्न
A 12.5 eV electron beam is used to bombard gaseous hydrogen at room temperature. What series of wavelengths will be emitted?
The first excited energy of a He+ ion is the same as the ground state energy of hydrogen. Is it always true that one of the energies of any hydrogen-like ion will be the same as the ground state energy of a hydrogen atom?
When white radiation is passed through a sample of hydrogen gas at room temperature, absorption lines are observed in Lyman series only. Explain.
What will be the energy corresponding to the first excited state of a hydrogen atom if the potential energy of the atom is taken to be 10 eV when the electron is widely separated from the proton? Can we still write En = E1/n2, or rn = a0 n2?
As one considers orbits with higher values of n in a hydrogen atom, the electric potential energy of the atom
Let An be the area enclosed by the nth orbit in a hydrogen atom. The graph of ln (An/A1) against ln(n)
(a) will pass through the origin
(b) will be a straight line with slope 4
(c) will be a monotonically increasing nonlinear curve
(d) will be a circle
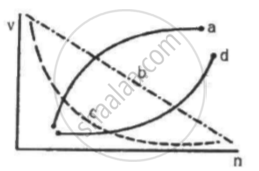
Find the binding energy of a hydrogen atom in the state n = 2.
A hydrogen atom emits ultraviolet radiation of wavelength 102.5 nm. What are the quantum numbers of the states involved in the transition?
A group of hydrogen atoms are prepared in n = 4 states. List the wavelength that are emitted as the atoms make transitions and return to n = 2 states.
Whenever a photon is emitted by hydrogen in Balmer series, it is followed by another photon in Lyman series. What wavelength does this latter photon correspond to?
Find the maximum angular speed of the electron of a hydrogen atom in a stationary orbit.
Suppose, in certain conditions only those transitions are allowed to hydrogen atoms in which the principal quantum number n changes by 2. (a) Find the smallest wavelength emitted by hydrogen. (b) List the wavelength emitted by hydrogen in the visible range (380 nm to 780 nm).
A hydrogen atom in ground state absorbs a photon of ultraviolet radiation of wavelength 50 nm. Assuming that the entire photon energy is taken up by the electron with what kinetic energy will the electron be ejected?
A hydrogen atom moving at speed υ collides with another hydrogen atom kept at rest. Find the minimum value of υ for which one of the atoms may get ionized.
The mass of a hydrogen atom = 1.67 × 10−27 kg.
Consider an excited hydrogen atom in state n moving with a velocity υ(ν<<c). It emits a photon in the direction of its motion and changes its state to a lower state m. Apply momentum and energy conservation principles to calculate the frequency ν of the emitted radiation. Compare this with the frequency ν0 emitted if the atom were at rest.
Positronium is just like a H-atom with the proton replaced by the positively charged anti-particle of the electron (called the positron which is as massive as the electron). What would be the ground state energy of positronium?
