Advertisements
Advertisements
प्रश्न
In the figure given below, LM = LN; angle PLN = 110o.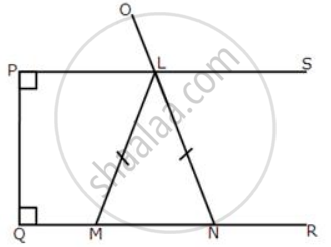
calculate: (i) ∠LMN
(ii) ∠MLN
उत्तर
Given: ∠PLN = 110°
(i) We know that the sum of the measure of all the angles of a quadrilateral is 360°.
In quad. PQNL,
∠QPL + ∠PLN + ∠LNQ + ∠NQP = 360°
⇒ 90° + 110° + ∠LNQ + 90° = 360°
⇒ ∠LNQ = 360° − 290°
⇒ ∠LNQ = 70°
⇒ ∠LNM = 70° ........(i)
In ΔLMN,
LM = LN ........( Given )
∴ ∠LNM = ∠LMN ....... [angles opp. to equal sides are equal]
⇒ ∠LMN = 70° ....(ii) [ from(i) ]
(ii) In ΔLMN,
∠LMN + ∠LNM+ ∠MLN = 180°
But ∠LNM= ∠LMN = 70° .....[ From(i) and (ii)]
∴ 70° + 70° + MLN = 180°
⇒ ∠MLN = 180°− 140°
⇒ ∠MLN = 40°
APPEARS IN
संबंधित प्रश्न
In the figure, given below, AB = AC.
Prove that: ∠BOC = ∠ACD.

In the given figure; AB = BC and AD = EC.
Prove that: BD = BE.
Prove that a triangle ABC is isosceles, if: altitude AD bisects angles BAC.
Prove that a triangle ABC is isosceles, if: bisector of angle BAC is perpendicular to base BC.
In the given figure, AD = AB = AC, BD is parallel to CA and angle ACB = 65°. Find angle DAC.
In isosceles triangle ABC, AB = AC. The side BA is produced to D such that BA = AD.
Prove that: ∠BCD = 90°
ABC is a triangle. The bisector of the angle BCA meets AB in X. A point Y lies on CX such that AX = AY.
Prove that: ∠CAY = ∠ABC.
Use the given figure to prove that, AB = AC.
Prove that the medians corresponding to equal sides of an isosceles triangle are equal.
The bisectors of the equal angles B and C of an isosceles triangle ABC meet at O. Prove that AO bisects angle A.
