Advertisements
Advertisements
प्रश्न
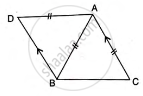
In the given figure, AD = AB = AC, BD is parallel to CA and angle ACB = 65°. Find angle DAC.
उत्तर
We can see that the ΔABC is an isosceles triangle with Side AB = Side AC.
⇒ ∠ACB = ∠ABC
As ∠ACB = 65°
hence ∠ABC = 65°
Sum of all the angles of a triangle is 180°
∠ACB + ∠CAB + ∠ABC = 180°
65°+ 65° + ∠CAB = 180°
∠CAB = 180° − 130°
∠CAB = 50°
As BD is parallel to CA
Therefore, ∠CAB = ∠DBA since they are alternate angles.
∠CAB = ∠DBA = 50°
We see that ΔADB is an isosceles triangle with Side AD = Side AB.
⇒ ∠ADB = ∠DBA = 50°
Sum of all the angles of a triangle is 180°
∠ADB + ∠DAB + ∠DBA = 180°
50° + ∠DAB + 50° = 180°
∠DAB = 180° − 100° = 80°
∠DAB = 80°
The angle DAC is the sum of angle DAB and CAB.
∠DAC = ∠CAB + ∠DAB
∠DAC = 50°+ 80°
∠DAC = 130°
APPEARS IN
संबंधित प्रश्न
In the figure given below, LM = LN; angle PLN = 110o.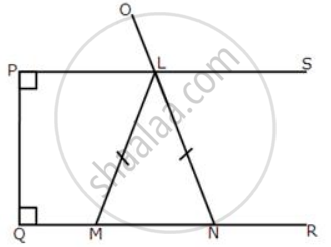
calculate: (i) ∠LMN
(ii) ∠MLN
In the figure, given below, AB = AC.
Prove that: ∠BOC = ∠ACD.

Calculate x :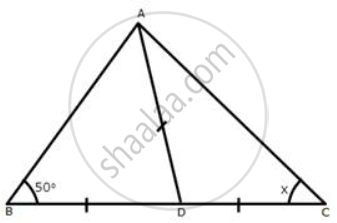
In the given figure; AB = BC and AD = EC.
Prove that: BD = BE.
Prove that a triangle ABC is isosceles, if: altitude AD bisects angles BAC.
Prove that a triangle ABC is isosceles, if: bisector of angle BAC is perpendicular to base BC.
In triangle ABC; AB = AC and ∠A : ∠B = 8 : 5; find angle A.
Using the information given of the following figure, find the values of a and b.

Prove that the medians corresponding to equal sides of an isosceles triangle are equal.
The bisectors of the equal angles B and C of an isosceles triangle ABC meet at O. Prove that AO bisects angle A.
