Question

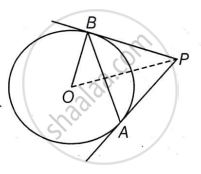
In the given figure, AB is chord of length 6 cm of a circle of radius 5 cm. The tangents at A and B intersect at point P. Find the length of PB.

Answer 1

Given AB = 6 cm and radius, OA = OB = 5 cm 

To find Length of PA

Construction Join OA, so OA = 5 cm

OL is perpendicular to AB.

AL = LB = `6/2 = 3`  ...[Since OL bisects AB]

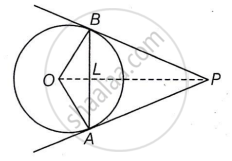
By Pythagoras theorem, in ΔOLA

OL² + LA² = OA²

∴ OL² = OA² − LA²

∴ OL² = 5² − 3²

∴ OL² = 25 − 9

∴ OL² = 16

∴ OL = 4 cm

We have, tan ∠AOL = `(AL)/(OL) = 3/4`

From ΔOAP,

tan ∠AOL = `(AL)/(OL)`

tan ∠AOP = `(PA)/(OA)`  ...[∵ ∠AOL = ∠AOP]

 `3/4 = (PA)/5`

∴ PA = `(3 xx 5)/4`

= `15/4`

= 3.75 cm

Length of PA = `15/4` = 3.75 cm