Advertisements
Advertisements
प्रश्न
In the given figure, PQ || AB; CQ = 4.8 cm QB = 3.6 cm and AB = 6.3 cm. Find :
- `(CP)/(PA)`
- PQ
- If AP = x, then the value of AC in terms of x.

उत्तर
i. In ΔCPQ and ΔCAB,
∠PCQ = ∠ACB ...(Since PQ || AB, so the angles are corresponding angles)
∠C = ∠C ...(Common angle)
∴ ΔCPQ ∼ ΔCAB ...(AA criterion for similarity)
`=> (CP)/(PA) = (CQ)/(QB)`
`=> (CP)/(PA) = (4.8)/(3.6) = (48)/(36) = 4/3`
So, `(CP)/(PA) = 4/3`
ii. In ΔCPQ and ΔCAB,
∠PCQ = ∠ACB ...(Since PQ || AB, so the angles are corresponding angles)
∠C = ∠C ...(Common angle)
∴ ΔCPQ ∼ ΔCAB ...(AA criterion for similarity)
`=> (PQ)/(AB) = (CQ)/(CB)`
`=> (PQ)/(6.3) = (4.8)/(8.4) `
So, PQ = 3.6
iii. In ΔCPQ and ΔCAB,
∠PCQ = ∠ACB ...(Since PQ || AB, so the angles are corresponding angles)
∠C = ∠C ...(Common angle)
∴ ΔCPQ ∼ ΔCAB ...(AA criterion for similarity)
`=> (CP)/(AC) = (CQ)/(CB)`
`=> (CP)/(AC) = (4.8)/(8.4) = 4/7 `
So, if AC is 7 parts, and CP is 4 parts, then PA is 3 parts.
Given, AP = x
or 3 parts = x
`=>` 1 part = `x/3`
`=>` 7 parts = `(7x)/3`
Hence, AC = `(7x)/3`
APPEARS IN
संबंधित प्रश्न
The perimeters of two similar triangles ABC and PQR are respectively 36 cm and 24 cm. If PQ = 10 cm, find AB
Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting AC in L and AD produced in E. Prove that EL = 2 BL
In the following figure, seg DH ⊥ seg EF and seg GK ⊥ seg EF. If DH = 18 cm, GK = 30 cm and `A(triangle DEF) = 450 cm^2`, then find:
1) EF
2) `A(triangle GFE)`
3) `A(square DFGE)`

In a right triangle ABC, right angled at B, D is a point on hypotenuse such that BD ⊥ AC , if DP ⊥ AB and DQ ⊥ BC then prove that
`(a) DQ^2 Dp.QC (b) DP ^2 DQ.AP 2 `

Are the triangles in the given figure similar? If yes, by which test?

Prove that, if a line is drawn parallel to one side of a triangle to intersect the other two sides, then the two sides are divided in the same ratio.
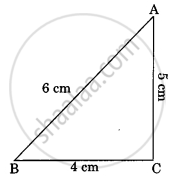
In the adjacent figure, ∆ACB ~ ∆APQ. If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm and AP = 2.8 cm, find CA and AQ

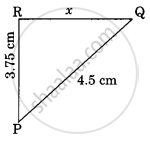
In the given figure ΔABC ~ ΔPQR. The value of x is
 |
 |
In the given figure, ∠ACB = ∠CDA, AC = 8cm, AD = 3cm, then BD is ______.

In ΔABC, PQ || BC. If PB = 6 cm, AP = 4 cm, AQ = 8 cm, find the length of AC.

