Advertisements
Advertisements
प्रश्न
In Young's double slit experiment, describe briefly how bright and dark fringes are obtained on the screen kept in front of a double slit. Hence obtain the expression for the fringe width.
Advertisements
उत्तर
In Young's double-slit experiment, the wavefronts from the two illuminated slits superpose on the screen. This leads to formation of alternate dark and bright fringes due to constructive and destructive interference, respectively. At the centre C of the screen, the intensity of light is maximum and it is called central maxima.
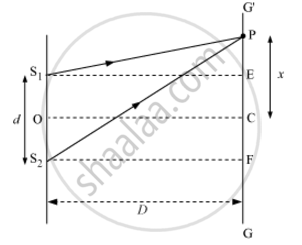
Let S1 and S2 be two slits separated by a distance d. GG'is the screen at a distance D from the slits S1 and S2. Point C is equidistant from both the slits. The intensity of light will be maximum at this point because the path difference of the waves reaching this point will be zero.
At point P, the path difference between the rays coming from the slits S1 and S2 is S2P - S1P.
Now, S1 S2 = d, EF = d, and S2F= D
∴In ΔS2PF,
`S_2P=[S_2F^2+PF^2]^(1/2)`
`S_2P=[D^2+(x+d/2)^2]^(1/2)`
`=D[1+(x+d/2)/D^2]^(1/2)`
Similarly, in ΔS1PE,
`S_1P=D[1+(x-d/2)^2/D^2]^(1/2)`
`:.S_2P-S_1P=D[1+1/2(x+d/2)^2/D^2]-D[1+1/2(x-d/2)^2/D^2]`
On expanding it binomially,
`S_2P-S_1P=1/(2D)[4xd/2]=(xd)/D`
For bright fringes (constructive interference), the path difference is an integral multiple of wavelengths, i.e. path difference is nλ.
`:.nlambda=(xd)/D`
`x=(nlambdaD)/d`where n = 0, 1, 2, 3, 4, …
For n = 0, x0 = 0
n =1, `x_1=(lambdaD)d`
n = 2, `x_2=(2lambdaD)/d`
n =3, `x_3=(3lambdaD)d`
`n=n, x_n = (nlambdaD)/d`
Fringe width (β) → Separation between the centres of two consecutive bright fringes is called the width of a dark fringe.
`:.beta_1=x_n-x_(n-1)=(lambdaD)/d`
Similarly, for dark fringes,
`x_n=(2n-1)lambda/2D/d`
For n =1, `x_1=(lambdaD)/(2d)`
For n =2, `x_2=(3lambdaD)/(2d)`
The separation between the centres of two consecutive dark interference fringes is the width of a bright fringe.
`:. beta_2=x_n-x_(n-1)=(lambdaD)/d`
∴β1 = β2
All the bright and dark fringes are of equal width as β1 = β2
APPEARS IN
संबंधित प्रश्न
Derive an expression for path difference in Young’s double slit experiment and obtain the conditions for constructive and destructive interference at a point on the screen.
In a Young’s double-slit experiment, the slits are separated by 0.28 mm and the screen is placed 1.4 m away. The distance between the central bright fringe and the fourth bright fringe is measured to be 1.2 cm. Determine the wavelength of light used in the experiment.
In Young's double slit experiment, derive the condition for
(i) constructive interference and
(ii) destructive interference at a point on the screen.
The intensity at the central maxima in Young’s double slit experimental set-up is I0. Show that the intensity at a point where the path difference is λ/3 is I0/4.
White light is used in a Young's double slit experiment. Find the minimum order of the violet fringe \[\left( \lambda = 400\text{ nm} \right)\] which overlaps with a red fringe \[\left( \lambda = 700\text{ nm} \right).\]
In Young's double slit experiment, the minimum amplitude is obtained when the phase difference of super-imposing waves is: (where n = 1, 2, 3, ...)
In a Young’s double slit experiment, the path difference at a certain point on the screen between two interfering waves is `1/8`th of the wavelength. The ratio of intensity at this point to that at the centre of a bright fringe is close to ______.
How will the interference pattern in Young's double-slit experiment be affected if the screen is moved away from the plane of the slits?
In Young's double slit experiment using light of wavelength 600 nm, the slit separation is 0.8 mm and the screen is kept 1.6 m from the plane of the slits. Calculate
- the fringe width
- the distance of (a) third minimum and (b) fifth maximum, from the central maximum.
In Young's double slit experiment the two slits are 0.6 mm distance apart. Interference pattern is observed on a screen at a distance 80 cm from the slits. The first dark fringe is observed on the screen directly opposite to one of the slits. The wavelength of light will be ______ nm.
