Advertisements
Advertisements
प्रश्न
Length of tape required to cover the edges of a semicircular disc of radius 10 cm is ______.
विकल्प
62.8 cm
51.4 cm
31.4 cm
15.7 cm
उत्तर
Length of tape required to cover the edges of a semicircular disc of radius 10 cm is 51.4 cm.
Explanation:
In order to find the length of tape required to cover the edges of a semi-circular disc, we have to find the perimeter of semi-circle

From the above figure it is clear that,
Perimeter of semi-circle = Circumference of semi-circle + Diameter
∵ Circumference of semi-Circle = `(2πr)/2`
= π × r
= `22/7 xx 10`
= `220/7`
= 31.4 cm
∴ Total tape required = 31.4 + 2 × 10 ......[∵ Diameter = 2 × radius]
= 51.4 cm
APPEARS IN
संबंधित प्रश्न
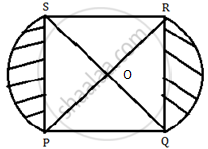
In the figure given below, PQRS is square lawn with side PQ = 42 metres. Two circular flower beds are there on the sides PS and QR with centre at O, the intersections of its
diagonals. Find the total area of the two flower beds (shaded parts).
A race track is in the form of a ring whose inner circumference is 352 m, and the outer circumference is 396 m. Find the width of the track.
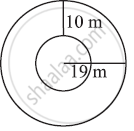
Find the circumference of the inner and the outer circles, shown in the adjoining figure? (Take π = 3.14)
Find the area of a right – angled triangle, the radius of whose, circumference measures 8 cm and the altitude drawn to the hypotenuse measures 6 cm.
A wire when bent in the form of a square encloses an area of 484cm2. If the same wire is bent into the form of a circle, find the area of the circle.
A Rose garden is in the form of circle of radius 63 m. The gardener wants to fence it at the rate of ₹ 150 per metre. Find the cost of fencing?
The cost of decorating the circumference of a circular lawn of a house at the rate of ₹ 55 per metre is ₹ 16940. What is the radius of the lawn?
Find the radius of a circle whose circumference is equal to the sum of the circumferences of two circles of radii 15 cm and 18 cm.
A circular pond is 17.5 m is of diameter. It is surrounded by a 2 m wide path. Find the cost of constructing the path at the rate of Rs 25 per m2.
The area of a semicircle of radius 4r is ______.
