Advertisements
Advertisements
प्रश्न
One angle of a hexagon is 140° and the remaining angles are in the ratio 4 : 3 : 4 : 5 : 4. Calculate the measures of the smallest and the largest angles.
उत्तर
A hexagon has 6 sides
∴ Sum of interior angles
= (n - 2) x 180°
= (6- 2) x 180°
= 4 x 180° = 720°
One angle is given to be 140°
Ratio of the remaining five angles
= 4 : 3 : 4 : 5: 4
∴ The interior angles are 4x°, 3x°, 4x°, 5x° and 4x°
∴ 140° + 4x° + 3x° + 4x° + 5° + 4x° = 720°
⇒ 20x° + 140° = 720°
⇒ x° = `(580°)/(20)` = 29°
The smallest angle is 3x° = 3.29° = 87°
The largest angle is 5x° = 5.29° = 145°.
APPEARS IN
संबंधित प्रश्न
ABCD is a parallelogram, AD is produced to E so that DE = DC and EC produced meets AB produced in F. Prove that BF = BC.
Complete of the following, so as to make a true statement:
A quadrilateral has ....... sides.
Complete of the following, so as to make a true statement:
A quadrilateral has ..... vertices, no three of which are .....
PQRSTU is a regular hexagon. Determine each angle of ΔPQT.
D and E are the mid-points of the sides AB and AC respectively of ∆ABC. DE is produced to F. To prove that CF is equal and parallel to DA, we need an additional information which is ______.
A quadrilateral can have all four angles as obtuse.
In given figure, name any four angles that appear to be acute angles.
What conclusion can be drawn from part of given figure, if BD bisects ∠ABC?
An angle is said to be trisected, if it is divided into three equal parts. If in the given figure, ∠BAC = ∠CAD = ∠DAE, how many trisectors are there for ∠BAE?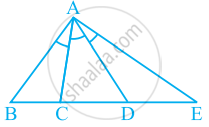
Draw a rough sketch of a quadrilateral PQRS. Draw its diagonals. Name them. Is the meeting point of the diagonals in the interior or exterior of the quadrilateral?