Advertisements
Advertisements
प्रश्न
A particle is fired vertically upward with a speed of 15 km s−1. With what speed will it move in interstellar space. Assume only earth's gravitational field.
उत्तर
Initial velocity of the particle, v = 15 km/s
Let its speed be v' in interstellar space.
Applying the law of conservation of energy, we have:
\[\left( \frac{1}{2} \right)m\left[ v - v '^2 \right] = \int_R^\infty \frac{GMm}{x^2}dx\]
\[ \therefore \left( \frac{1}{2} \right)m\left[ 15 \times {10}^3 - v '^2 \right] = \int_R^\infty \frac{GMm}{x^2}dx\]
\[ \Rightarrow \left( \frac{1}{2} \right)m\left[ \left( 15 \times {10}^3 \right)^2 - v '^2 \right] = GMm\left[ \frac{- 1}{x} \right]\]
\[ \Rightarrow \left( \frac{1}{2} \right)m\left[ \left( 225 \times {10}^5 \right) - v '^2 \right] = \frac{GMm}{R}\]
\[ \Rightarrow 225 \times {10}^5 - v '^2 = \frac{2 \times 6 . 67 \times {10}^{- 11} \times 6 \times {10}^{24}}{6400 \times {10}^3}\]
\[ \Rightarrow v '^2 = 225 \times {10}^6 - \frac{40 . 02}{32} \times {10}^8 \]
\[ = 2 . 25 \times {10}^8 - 1 . 2 \times {10}^8 \]
\[ = {10}^8 \left( 1 . 05 \right)\]
\[\text { Or }\ v' = 1 . 01 \times {10}^4 m/s = 10 km/s\]
APPEARS IN
संबंधित प्रश्न
An apple falls from a tree. An insect in the apple finds that the earth is falling towards it with an acceleration g. Who exerts the force needed to accelerate the earth with this acceleration g?
The acceleration of moon with respect to earth is 0⋅0027 m s−2 and the acceleration of an apple falling on earth' surface is about 10 m s−2. Assume that the radius of the moon is one fourth of the earth's radius. If the moon is stopped for an instant and then released, it will fall towards the earth. The initial acceleration of the moon towards the earth will be
The acceleration of the moon just before it strikes the earth in the previous question is
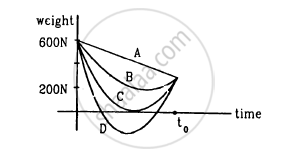
Suppose, the acceleration due to gravity at the earth's surface is 10 m s−2 and at the surface of Mars it is 4⋅0 m s−2. A 60 kg passenger goes from the earth to the Mars in a spaceship moving with a constant velocity. Neglect all other objects in the sky. Which part of the following figure best represents the weight (net gravitational force) of the passenger as a function of time?
Find the height over the Earth's surface at which the weight of a body becomes half of its value at the surface.
A body is weighed by a spring balance to be 1.000 kg at the North Pole. How much will it weigh at the equator? Account for the earth's rotation only.
A particle is fired vertically upward from earth's surface and it goes up to a maximum height of 6400 km. Find the initial speed of particle.
If the acceleration due to gravity becomes 4 times its original value, then escape speed ____________.
Suppose we go 200 km above and below the surface of the Earth, what are the g values at these two points? In which case, is the value of g small?
One can easily weigh the earth by calculating the mass of the earth by using the formula:
The earth is an approximate sphere. If the interior contained matter which is not of the same density everywhere, then on the surface of the earth, the acceleration due to gravity ______.
Which of the following options are correct?
- Acceleration due to gravity decreases with increasing altitude.
- Acceleration due to gravity increases with increasing depth (assume the earth to be a sphere of uniform density).
- Acceleration due to gravity increases with increasing latitude.
- Acceleration due to gravity is independent of the mass of the earth.
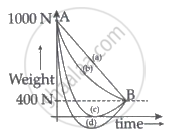
A person whose mass is 100 kg travels from Earth to Mars in a spaceship. Neglect all other objects in the sky and take acceleration due to gravity on the surface of the Earth and Mars as 10 m/s2 and 4 m/s2 respectively. Identify from the below figures, the curve that fits best for the weight of the passenger as a function of time.
A ball is immersed in water kept in container and released. At the same time container is accelerated in horizontal direction with acceleration, `sqrt44` m/s2. Acceleration of ball w.r.t. container is ______ m/s2 (specific gravity of ball = 12/17, g = 10 m/s2)
