Advertisements
Advertisements
प्रश्न
A mass of 6 × 1024 kg (equal to the mass of the earth) is to be compressed in a sphere in such a way that the escape velocity from its surface is 3 × 108 m s−1. What should be the radius of the sphere?
उत्तर
Mass of the sphere = 6 × 1024 kg
Escape velocity = 3 × 108 m/s
Escape velocity is given by
\[v_e = \frac{2GM}{R}\]
\[ \Rightarrow R = \frac{2GM}{{v_e}^2}\]
\[ = \frac{2 \times 6 . 67 \times {10}^{- 11} \times 6 \times {10}^{24}}{\left( 3 \times {10}^8 \right)^2}\]
\[ = \frac{2 \times 40 . 02 \times {10}^{13}}{9 \times {10}^{16}}\]
\[ = \frac{80 . 02}{9} \times {10}^{- 3} m\]
\[ = 8 . 89 \times {10}^{- 3} m\]
\[ = 9 \ \text { mm }\]
APPEARS IN
संबंधित प्रश्न
Assuming the earth to be a sphere of uniform mass density, how much would a body weigh half way down to the centre of the earth if it weighed 250 N on the surface?
If heavier bodies are attracted more strongly by the earth, why don't they fall faster than the lighter bodies?
An apple falls from a tree. An insect in the apple finds that the earth is falling towards it with an acceleration g. Who exerts the force needed to accelerate the earth with this acceleration g?
Suppose, the acceleration due to gravity at the earth's surface is 10 m s−2 and at the surface of Mars it is 4⋅0 m s−2. A 60 kg passenger goes from the earth to the Mars in a spaceship moving with a constant velocity. Neglect all other objects in the sky. Which part of the following figure best represents the weight (net gravitational force) of the passenger as a function of time?
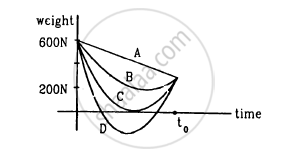
Take the effect of bulging of earth and its rotation in account. Consider the following statements :
(A) There are points outside the earth where the value of g is equal to its value at the equator.
(B) There are points outside the earth where the value of g is equal to its value at the poles.
Find the height over the Earth's surface at which the weight of a body becomes half of its value at the surface.
Find the acceleration due to gravity in a mine of depth 640 m if the value at the surface is 9.800 m s−2. The radius of the earth is 6400 km.
A body is weighed by a spring balance to be 1.000 kg at the North Pole. How much will it weigh at the equator? Account for the earth's rotation only.
A particle is fired vertically upward from earth's surface and it goes up to a maximum height of 6400 km. Find the initial speed of particle.
If the acceleration due to gravity becomes 4 times its original value, then escape speed ____________.
Explain the variation of g with altitude.
Suppose we go 200 km above and below the surface of the Earth, what are the g values at these two points? In which case, is the value of g small?
If both the mass and the radius of the earth decrease by 1%, then the value of acceleration due to gravity will
The earth is an approximate sphere. If the interior contained matter which is not of the same density everywhere, then on the surface of the earth, the acceleration due to gravity ______.
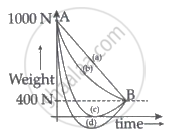
A person whose mass is 100 kg travels from Earth to Mars in a spaceship. Neglect all other objects in the sky and take acceleration due to gravity on the surface of the Earth and Mars as 10 m/s2 and 4 m/s2 respectively. Identify from the below figures, the curve that fits best for the weight of the passenger as a function of time.
A ball is immersed in water kept in container and released. At the same time container is accelerated in horizontal direction with acceleration, `sqrt44` m/s2. Acceleration of ball w.r.t. container is ______ m/s2 (specific gravity of ball = 12/17, g = 10 m/s2)
