Advertisements
Advertisements
प्रश्न
Perimeter of a parallelogram shaped land is 96 m and its area is 270 square metres. If one of the sides of this parallelogram is 18 m, find the length of the other side. Also, find the lengths of altitudes l and m (see figure).
Advertisements
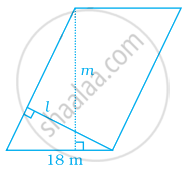
उत्तर
Given, perimeter of parallelogram = 96 m and area of parallelogram = 270 m2
In a parallelogram ABCD, AB = CD = 18 m and AD = BC
As we know, perimeter of a parallelogram ABCD = AB + BC + CD + AD
⇒ 96 = 18 + AD + 18 + AD ...[∵ AD = BC]
⇒ 96 = 36 + 24D
⇒ 2AD = 60
⇒ AD = 30 cm
So, AD = BC = 30 cm
Now, area of parallelogram ABCD = Base × Corresponding height
⇒ 270 = AB × DE ...[∵ Base = AB]
⇒ 270 = 18 × DE
⇒ `270/18` = DE
⇒ DE = 15 m
Also, area of parallelogram ABCD = AD × BF ...[∵ Base = AD]
⇒ 270 = 30 × l
⇒ l = `270/30`
⇒ l = 9 m
Hence, altitudes l = 9 m and m = 15 m.
APPEARS IN
संबंधित प्रश्न
Find the area of the following parallelogram:
PQRS is a parallelogram (see the given figure). QM is the height from Q to SR and QN is the height from Q to PS. If SR = 12 cm and QM = 7.6 cm. Find:
- the area of the parallelogram PQRS
- QN, if PS = 8 cm
If area of a parallelogram is 29.6 sq cm and its base is 8 cm, find its height.
A parallelogram has sides of 15 cm and 12 cm; if the distance between the 15 cm sides is 6 cm; find the distance between 12 cm sides.
Find the missing values.
| Base | Height | Area |
| 18 cm | 5 cm |
Find the missing value:
| Base | Height | Area of parallelogram |
| 15.6 cm | ______ | 16.38 cm2 |
Observe the shapes 1, 2, 3 and 4 in the figures. Which of the following statements is not correct?
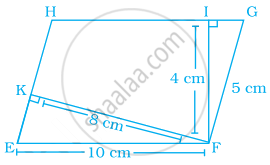
In the following figure, EFGH is a parallelogram, altitudes FK and FI are 8 cm and 4 cm respectively. If EF = 10 cm, then area of EFGH is ______.
Perpendicular dropped on the base of a parallelogram from the opposite vertex is known as the corresponding ______ of the base.
In the following figure, find the area of parallelogram ABCD if the area of shaded triangle is 9 cm2.
