Advertisements
Advertisements
प्रश्न
Prove that for an astroid ` x^(2/3) +y2/3= a^(2/3)` the line 𝜽=𝝅/𝟔 Divide the arc in the first quadrant in a ratio 1:3.
उत्तर
Given curve : astroid` x^(2/3)+y^(2/3) = a^(2/3)`
The line 𝜽=𝝅/𝟔 cuts the asroid in 1 st quadrant.
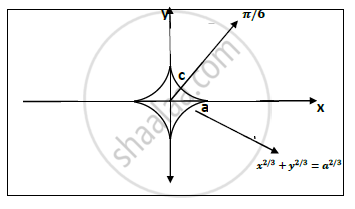
C is the point on the curve which cuts the arc.
Length of astroid in first quadrant:
Put ` x = acos^3t and y=asin^3t`
`dx=-3 asin t.cos^2tdt dy=3 acos t.sin^2tdt`
`s= int_0^(pi/2) sqrt ((dx/dt)^2+(dy/dt)^2)=int_0^(pi/2) sqrt((-3asin t.cos^2 t)^2+(3 acos t .sin^2 t )^2)` dt
= `int_0^(pi/2) 3a.sin t.cost dt`
= `3/2 a int_0^(pi/2) sin 2t dt`
=`3/4 a [-cos 2t ]_0^(pi/2) `
∴ `s= 3/2 a ` ………………….(1)
Now the length of the curve ac : Just put `pi/6` 𝒊𝒏𝒔𝒕𝒆𝒅 𝒐𝒇 `pi/2` because the curve is Only upto given line.
∴ S(ac) =`int_0^(pi/6) 3a sint . cost dt =3/4a [-cos 2t]_0^(pi/6) `
=` 3/4 a [-1/2+1]`
`s(ac)= 3/8 a ` ……………(2)
Legnth of remaining part = `3/2a-3/8 a=9/8 a` ……………….(3)
Divide eqn (3) and (2).
The line `pi/6` cuts the given astroid in the ratio of 1:3
Hence proved.
