Question

Prove that the line segment joining the point of contact of two parallel tangents to a circle is a diameter of the circle.

Answer 1

Given: CD and EF are two parallel tangents at the points A and B of a circle with centre O.

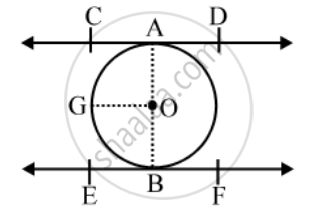
To prove: AOB is a diameter of the circle.

Construction: Join OA and OB.

Draw OG || CD

Proof: OG || CD and AO cuts them.

∴ ∠CAO + ∠GOA = 180°

⇒ ∠GOA = 180°

⇒ ∠GOA = 90°

Similarly, ∠GOB = 90°

∴ ∠GOA + ∠GOB = (90° + 90°) = 180°

⇒ AOB is a straight line

Hence, AOB is a diameter of the circle with centre O.