Advertisements
Advertisements
प्रश्न
Represent the following statement by the Venn diagram.
Some non-resident Indians are not rich.
उत्तर
Let, U : The set of all human beings.
N : The set of all non-resident Indians.
R : The set of all rich people.
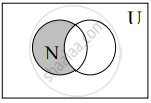
The above Venn diagram represents the truth of the given statement i.e., N - R ≠ φ
APPEARS IN
संबंधित प्रश्न
Draw appropriate Venn diagram for the following:
(A ∪ B)'
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find\[B \cup D\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[A \cap C\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[A \cap D\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[B \cap C\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[B \cap D\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}. Find: \[A - C\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}. Find: \[C - A\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}.
Find: \[D - A\]
Let U = {1, 2, 3, 4, 5, 6, 7, 8, 9}, A = {2, 4, 6, 8} and B = {2, 3, 5, 7}. Verify that \[\left( A \cup B \right)' = A' \cap B'\]
Represent the union of two sets by Venn diagram for the following.
P = {a, b, c, e, f} Q = {l, m, n, e, b}
Express the truth of the following statements with the help of Venn diagram:
(a) No circles are polygon
(b) If a quadrilateral is rhombus , then it is a parallelogram .
Using the Venn diagram, examine the logical equivalence of the following statements:
(a) Some politicians are actors.
(b) There are politicians who are actors.
(c) There are politicians who are not actors.
Express the truth of each of the following statements using Venn diagram.
(1) All teachers are scholars and scholars are teachers.
(2) If a quadrilateral is a rhombus then it is a parallelogram..
From the given diagram find :
A' ∩ B
Use the given Venn-diagram to find:
B - A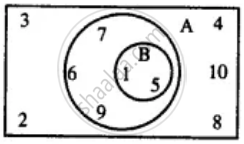
Use the given Venn-diagram to find :
B'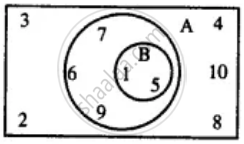
Draw a Venn-diagram to show the relationship between two overlapping sets A and B. Now shade the region representing :
A ∩ B
Draw a Venn-diagram to show the relationship between two overlapping sets A and B. Now shade the region representing :
A ∪ B
Draw a Venn-diagram to show the relationship between two sets A and B; such that A ⊆ B, Now shade the region representing :
A ∪ B
Two sets A and B are such that A ∩ B = Φ. Draw a venn-diagram to show the relationship between A and B. Shade the region representing :
(A ∪ B)'
Two sets A and B are such that A ∩ B = Φ. Draw a venn-diagram to show the relationship between A and B. Shade the region representing :
B - A
State the sets representing by the shaded portion of following venn-diagram :
In the given diagram, shade the region which represents the set given underneath the diagrams: (A ∩ B)'
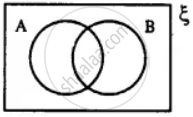
Using the given diagram, express the following sets in the terms of A and B. {a, d}
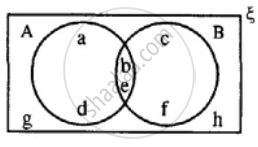
Using the given diagram, express the following sets in the terms of A and B. {a, d, c, f}
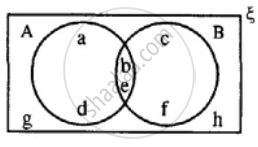
Represent the truth of the following statement by the Venn diagram.
Some hardworking students are obedient.
Represent the truth of the following statement by the Venn diagram.
No circles are polygons.
Represent the truth of the following statement by the Venn diagram.
If a quadrilateral is a rhombus, then it is a parallelogram.
Draw a Venn diagram for the truth of the following statement.
Some share brokers are chartered accountants.
Express the truth of the following statement by the Venn diagram.
Some persons are not politician.
Draw the Venn diagrams to illustrate the following relationship among sets E, M and U, where E is the set of students studying English in a school, M is the set of students studying Mathematics in the same school, U is the set of all students in that school.
All the students who study Mathematics study English, but some students who study English do not study Mathematics.
Draw the Venn diagrams to illustrate the following relationship among sets E, M and U, where E is the set of students studying English in a school, M is the set of students studying Mathematics in the same school, U is the set of all students in that school.
There is no student who studies both Mathematics and English.
Draw the Venn diagrams to illustrate the following relationship among sets E, M and U, where E is the set of students studying English in a school, M is the set of students studying Mathematics in the same school, U is the set of all students in that school.
Not all students study Mathematics, but every students studying English studies Mathematics.
Let U be the set of all boys and girls in a school, G be the set of all girls in the school, B be the set of all boys in the school, and S be the set of all students in the school who take swimming. Some, but not all, students in the school take swimming. Draw a Venn diagram showing one of the possible interrelationship among sets U, G, B and S.
