Advertisements
Advertisements
प्रश्न
Show by means of graphical method that: v = u + at, where the symbols have their usual meanings.
Advertisements
उत्तर
Consider the velocity-time graph of a body shown in figure.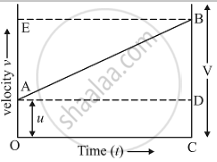
The body has an initial velocity (u) at a point A and then, its velocity changes at a uniform rate from A to B in time, (t). In other words, there is a uniform acceleration (a) from A to B, and after time (t) its final velocity becomes (v) which is equal to BC in the graph. The time (t) is represented by OC. To complete the figure, we draw the perpendicular CB from point C, and draw AD parallel to OC. BE is the perpendicular from point B to OE.
And, final velocity of the body, (v) = BC …… (2)
But from the graph BC = BD + DC
Therefore, v = BD + DC ……(3)
Again DC = OA
So, v = BD + OA
Now, from equation (1),
So, v = BD + u ......(4)
We should find out the value of the slope of a velocity-time graph which is equal to the acceleration,
Thus, Acceleration,
`a = "BD"/"AD"`
But AD = OC = t, so putting t in place of AD in the above relation, we get,
`a = "BD"/t`
So, BD = at
Now, putting this value of BD in equation (4), we get the 1st equation of motion-:
`v = u + at`
where,
(a) - Acceleration
(v) - Final velocity
(u) - Initial velocity
(t) - Time taken
APPEARS IN
संबंधित प्रश्न
The velocity-time graph for part of a train journey is a horizontal straight line. What does this tell you about its acceleration ?
Three speed-time graphs are given below :
Which graph represents the case of:
(i) a cricket ball thrown vertically upwards and returning to the hands of the thrower ?
(ii) a trolley decelerating to a constant speed and then accelerating uniformly ?
Draw velocity – time graph for the following situation:
When a body, is moving with uniform velocity.
From the velocity – time graph given below, calculate Distance covered in the region ABCE.
Diagram is given below shows velocity – time graph of car P and Q, starting from the same place and in the same direction. Calculate at what time intervals both cars have the same velocity?
Draw the speed-time graph of a body starting from some point P, gradually picking up speed, then running at a uniform speed and finally slowing down to stop at some point Q.
Draw the following graph:
Speed versus time for a stepped motion
(i) In speed time graph uniform motion is given by a straight line parallel to x axis so figure (a) denotes the uniform motion.
(ii) In speed time graph motion with increasing speed is shown by straight line with positive slope so figure (c) denotes the motion with speed increasing.
(iii) In speed time graph motion with decreasing speed is shown by straight line with negative slope so figure (b) denotes the motion with speed decreasing.
(iv) In speed time graph motion with oscillating speed is shown by zigzag line so figure {d) denotes the motion with speed oscillating.
The slope of the speed–time graph gives ______.
If the velocity-time graph has the shape AMB, what would be the shape of the corresponding acceleration-time graph?
