Advertisements
Advertisements
प्रश्न
Solve 3x + 8 > 2, when x is a real number.
उत्तर
The given inequality is 3x + 8 > 2.
3x + 8 > 2
⇒ 3x + 8 - 8 > 2 - 8
⇒ 3x > - 6
⇒ `(3x)/3 > (-6)/3`
⇒ x > - 2
When x is a real number, the solutions of the given inequality are all the real numbers, which are greater than –2.
Thus, in this case, the solution set is (– 2, ∞).
APPEARS IN
संबंधित प्रश्न
Solve 24x < 100, when x is a natural number.
Solve 24x < 100, when x is an integer.
Solve –12x > 30, when x is a natural number.
Solve –12x > 30, when x is an integer.
Solve 5x – 3 < 7, when x is an integer.
Solve 5x – 3 < 7, when x is a real number.
Solve 3x + 8 > 2, when x is an integer.
Solve the given inequality for real x: 4x + 3 < 5x + 7.
Solve the given inequality for real x: 3(2 – x) ≥ 2(1 – x)
Solve the given inequality for real x: `(3(x-2))/5 <= (5(2-x))/3`
Solve the given inequality for real x: `1/2 ((3x)/5 + 4) >= 1/3 (x -6)`
Solve the given inequality for real x: 2(2x + 3) – 10 < 6 (x – 2)
Solve the given inequality and show the graph of the solution on number line:
3x – 2 < 2x +1
Find all pairs of consecutive even positive integers, both of which are larger than 5 such that their sum is less than 23.
A man wants to cut three lengths from a single piece of board of length 91 cm. The second length is to be 3 cm longer than the shortest and the third length is to be twice as long as the shortest. What are the possible lengths of the shortest board if the third piece is to be at least 5 cm longer than the second?
[Hint: If x is the length of the shortest board, then x, (x + 3) and 2x are the lengths of the second and third piece, respectively. Thus, x = (x + 3) + 2x ≤ 91 and 2x ≥ (x + 3) + 5]
Solve the inequality.
6 ≤ –3(2x – 4) < 12
Solve the inequality.
`-3 <= 4 - (7x)/2 <= 18`
Solve the inequality.
`7 <= (3x + 11)/2 <= 11`
Represent to solution set of each of the following inequations graphically in two dimensional plane:
x + 2y − y ≤ 0
Represent to solution set of each of the following inequations graphically in two dimensional plane:
x + 2 ≥ 0
Represent to solution set of each of the following inequations graphically in two dimensional plane:
0 ≤ 2x − 5y + 10
Represent to solution set of each of the following inequations graphically in two dimensional plane:
3y ≥ 6 − 2x
Represent to solution set of each of the following inequations graphically in two dimensional plane:
y ≥ 2x − 8
Represent to solution set of each of the following inequations graphically in two dimensional plane:
3x − 2y ≤ x + y − 8
Solutions of the inequalities comprising a system in variable x are represented on number lines as given below, then ______.![]()
State whether the following statement is True or False.
If xy < 0, then x > 0, and y > 0
The inequality representing the following graph is ______.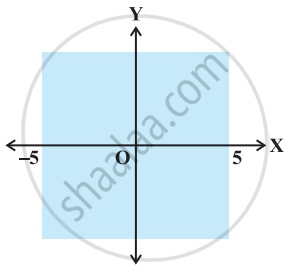
Solution of a linear inequality in variable x is represented on number line given below ______.
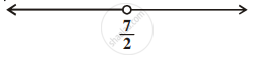
Solution of a linear inequality in variable x is represented on number line given below ______.
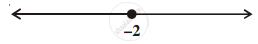
If x > –2 and x < 9, then x ∈ (– 2, 9)
If |x| > 5, then x ∈ (– `oo`, – 5) ∪ [5, `oo`)
