Advertisements
Advertisements
प्रश्न
Suppose we have 12 protons and 12 neutrons. We can assemble them to form either a 24Mg nucleus or two 12C nuclei. In which of the two cases more energy will be liberated?
उत्तर
If we assemble 6 protons and 6 neutrons to form 12C nucleus, 92.15 MeV (product of mass number and binding energy per nucleon of carbon-12) of energy is released. Therefore, the energy released in the formation of two carbon nuclei is 184.3 MeV. On the other hand, when 12 protons and 12 neutrons are combined to form a 24Mg atom, 198.25 MeV of energy (binding energy) is released. Hence, in case of 24Mg nucleus, more energy is liberated.
APPEARS IN
संबंधित प्रश्न
Draw the plot of binding energy per nucleon (BE/A) as a functino of mass number A. Write two important conclusions that can be drawn regarding the nature of nuclear force.
Using the curve for the binding energy per nucleon as a function of mass number A, state clearly how the release in energy in the processes of nuclear fission and nuclear fusion can be explained.
A heavy nucleus X of mass number 240 and binding energy per nucleon 7.6 MeV is split into two fragments Y and Z of mass numbers 110 and 130. The binding energy of nucleons in Y and Z is 8.5 MeV per nucleon. Calculate the energy Q released per fission in MeV.
The mass number of a nucleus is equal to
Which of the following is a wrong description of binding energy of a nucleus?
In one average-life,
For nuclei with A > 100,
(a) the binding energy of the nucleus decreases on an average as A increases
(b) the binding energy per nucleon decreases on an average as A increases
(c) if the nucleus breaks into two roughly equal parts, energy is released
(d) if two nuclei fuse to form a bigger nucleus, energy is released.
Assume that the mass of a nucleus is approximately given by M = Amp where A is the mass number. Estimate the density of matter in kgm−3 inside a nucleus. What is the specific gravity of nuclear matter?
A neutron star has a density equal to that of the nuclear matter. Assuming the star to be spherical, find the radius of a neutron star whose mass is 4.0 × 1030 kg (twice the mass of the sun).
Calculate the mass of an α-particle. Its Its binding energy is 28.2 MeV.
(Use Mass of proton mp = 1.007276 u, Mass of `""_1^1"H"` atom = 1.007825 u, Mass of neutron mn = 1.008665 u, Mass of electron = 0.0005486 u ≈ 511 keV/c2,1 u = 931 MeV/c2.)
(a) Calculate the energy released if 238U emits an α-particle. (b) Calculate the energy to be supplied to 238U it two protons and two neutrons are to be emitted one by one. The atomic masses of 238U, 234Th and 4He are 238.0508 u, 234.04363 u and 4.00260 u respectively.
(Use Mass of proton mp = 1.007276 u, Mass of `""_1^1"H"` atom = 1.007825 u, Mass of neutron mn = 1.008665 u, Mass of electron = 0.0005486 u ≈ 511 keV/c2,1 u = 931 MeV/c2.)
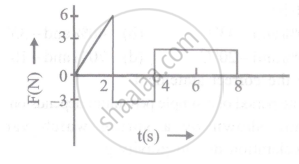
The force 'F' acting on a particle of mass 'm' is indicated by the force-time graph shown below. The change in momentum of the particle over the time interval from zero to 8s is:
A nucleus of mass M emits a γ-ray photon of frequency 'v'. The loss of internal energy by the nucleus is ______.
