Advertisements
Advertisements
प्रश्न
The bisectors of the angle of a parallelogram enclose a
विकल्प
parallelogram
rhombus
rectangle
square
उत्तर
We have ABCD, a parallelogram given below:

Therefore, we have AB || BC
Now, AD || BC and transversal AB intersects them at A and B respectively. Therefore,
Sum of consecutive interior angle is supplementary. That is;
∠A + ∠B = 180°
`1/2∠A + 1/2∠B = 90°`
We have AR and BR as bisectors of ∠A and ∠B respectively.
∠RAB +∠RBA = 90° …… (i)
Now, in ΔABR, by angle sum property of a triangle, we get:
∠RAB + ∠RBA +∠ARB = 180°
From equation (i), we get:
90° + ∠ARB = 180°
∠ARB = 90°
Similarly, we can prove that ∠DPC = 90° .
Now, AB || DC and transversal ADintersects them at A and D respectively. Therefore,
Sum of consecutive interior angle is supplementary. That is;
∠A + ∠D = 180°
`1/2∠A+1/2 ∠D = 90°`
We have AR and DP as bisectors of ∠A and ∠D respectively.
∠DAR + ∠ADP = 90° …… (ii)
Now, in ΔADR, by angle sum property of a triangle, we get:
∠DAR + ∠ADP + ∠AQD = 180°
From equation (i), we get:
90° +∠AQD = 180°
∠AQD = 90°
We know that ∠AQD and ∠PQR are vertically opposite angles, thus,
∠PQR = 90°
Similarly, we can prove that ∠PSR = 90° .
Therefore, PQRS is a rectangle.
Hence, the correct choice is (c).
APPEARS IN
संबंधित प्रश्न
Show that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.
ABCD is a rhombus. Show that diagonal AC bisects ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.
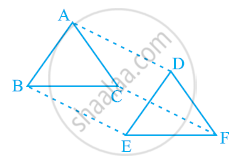
In ΔABC and ΔDEF, AB = DE, AB || DE, BC = EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see the given figure). Show that
(i) Quadrilateral ABED is a parallelogram
(ii) Quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) Quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) ΔABC ≅ ΔDEF.
In a parallelogram ABCD, ∠D = 135°, determine the measures of ∠A and ∠B
The following statement are true and false .
If three sides of a quadrilateral are equal, it is a parallelogram .
The following statement are true and false .
If three angles of a quadrilateral are equal, it is a parallelogram .
The figure formed by joining the mid-points of the adjacent sides of a rectangle is a
If one angle of a parallelogram is 24° less than twice the smallest angle, then the measure of the largest angle of the parallelogram is
Diagonals of a quadrilateral ABCD bisect each other. If ∠A= 45°, then ∠B =
ABCD is a parallelogram and E is the mid-point of BC. DE and AB when produced meet at F. Then, AF =
