Advertisements
Advertisements
प्रश्न
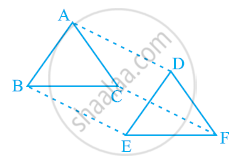
In ΔABC and ΔDEF, AB = DE, AB || DE, BC = EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see the given figure). Show that
(i) Quadrilateral ABED is a parallelogram
(ii) Quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) Quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) ΔABC ≅ ΔDEF.
उत्तर
(i) It is given that AB = DE and AB || DE.
If two opposite sides of a quadrilateral are equal and parallel to each other, then it will be a parallelogram.
Therefore, quadrilateral ABED is a parallelogram.
(ii) Again, BC = EF and BC || EF
Therefore, quadrilateral BCEF is a parallelogram.
(iii) As we had observed that ABED and BEFC are parallelograms, therefore
AD = BE and AD || BE
(Opposite sides of a parallelogram are equal and parallel)
And, BE = CF and BE || CF
(Opposite sides of a parallelogram are equal and parallel)
∴ AD = CF and AD || CF
(iv) As we had observed that one pair of opposite sides (AD and CF) of quadrilateral ACFD are equal and parallel to each other, therefore, it is a parallelogram.
(v) As ACFD is a parallelogram, therefore, the pair of opposite sides will be equal and parallel to each other.
∴ AC || DF and AC = DF
(vi) ΔABC and ΔDEF,
AB = DE (Given)
BC = EF (Given)
AC = DF (ACFD is a parallelogram)
∴ ΔABC ≅ ΔDEF (By SSS congruence rule)
APPEARS IN
संबंधित प्रश्न
In a parallelogram ABCD, ∠D = 135°, determine the measures of ∠A and ∠B
The following statement are true and false .
In a parallelogram, the diagonals intersect each other at right angles .
The following statement are true and false .
If three sides of a quadrilateral are equal, it is a parallelogram .
The following statement are true and false .
If three angles of a quadrilateral are equal, it is a parallelogram .
The perimeter of a parallelogram is 22 cm. If the longer side measures 6.5 cm, what is the measure of shorter side?
Complete the following statement by means of one of those given in brackets against each:
Consecutive angles of a parallelogram are ...................
The bisectors of any two adjacent angles of a parallelogram intersect at
The bisectors of the angle of a parallelogram enclose a
The figure formed by joining the mid-points of the adjacent sides of a quadrilateral is a
The diagonals of a parallelogram ABCD intersect at O. If ∠BOC = 90° and ∠BDC = 50°, then ∠OAB =
