Advertisements
Advertisements
प्रश्न
The diagonals of a parallelogram ABCD intersect at O. If ∠BOC = 90° and ∠BDC = 50°, then ∠OAB =
विकल्प
40°
50°
10°
90°
उत्तर
ABCD is a parallelogram with diagonals AC and BD intersect at O.
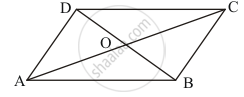
It is given that ∠BOC = 90° and∠BDC = 50°.
We need to find ∠OAB
Now,
∠BOC +∠COD = 180° (Linear pair)
90° + ∠COD = 180
∠COD = 90°
Since, O lies on BD.
Therefore,
∠ODC = ∠BDC
∠ODC = 50°
By angle sum property of a triangle, we get:
∠ODC + ∠COD + ∠OCD = 180°
50° + 90° + ∠OCD = 180°
140° + ∠OCD = 180°
∠OCD = 40°
Since, O lies on AC.
Therefore,
∠ACD = ∠OCD
∠ACD = 40°
Also, DC || AB
Therefore,
∠PAB = ∠ACD
∠OAB = 40°
Hence the correct choice is (a).
APPEARS IN
संबंधित प्रश्न
ABCD is a rhombus. Show that diagonal AC bisects ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.
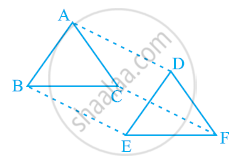
In ΔABC and ΔDEF, AB = DE, AB || DE, BC = EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see the given figure). Show that
(i) Quadrilateral ABED is a parallelogram
(ii) Quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) Quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) ΔABC ≅ ΔDEF.
The following statement are true and false.
In a parallelogram, the diagonals bisect each other.
The following statement are true and false .
If three sides of a quadrilateral are equal, it is a parallelogram .
The perimeter of a parallelogram is 22 cm. If the longer side measures 6.5 cm, what is the measure of shorter side?
Complete the following statement by means of one of those given in brackets against each:
If one angle of a parallelogram is a right angle, then it is necessarily a .................
PQRS is a quadrilateral, PR and QS intersect each other at O. In which of the following case, PQRS is a parallelogram?
PQ = 7 cm, QR = 7 cm, RS = 8 cm, SP = 8 cm
PQRS is a quadrilateral, PR and QS intersect each other at O. In which of the following case, PQRS is a parallelogram?
OP = 6.5 cm, OQ = 6.5 cm, OR = 5.2 cm, OS = 5.2 cm
The figure formed by joining the mid-points of the adjacent sides of a quadrilateral is a
ABCD is a parallelogram and E and F are the centroids of triangles ABD and BCDrespectively, then EF =
