Advertisements
Advertisements
प्रश्न
The difference between the sides at the right angles in a right-angled triangle is 7 cm. the area of the triangle is `60 cm^2` . Find its perimeter.
उत्तर
Area of the triangle 60cm^2
Let the sides of the triangle be a, b and c, where a is the height, b is the base and c is hypotenuse of the triangle.
`a-b=7cm`
`a=7+b` ....................(1)
Area of triangle `=1/2xxbxxh`
⇒`60=1/2xxbxx(7+b)`
⇒ `120=7b+b^2`
⇒`b^2+7b+b^2`
⇒`(b+15) (b-8)=0`
⇒`b=-15 or 8`
Side of a triangle cannot be negative.
Therefore, b = 8 cm.
Substituting the value of b = 8 cm, in equation (1):
`a=7+8=15 cm`
`Now, a=15 cm, b=8cm`
Now, in the given right triangle, we have to find third side.
`"(Hyp)"^2="(First side)"^2+"(Second side)"^2`
⇒` Hyp^2` =`8^2+15^2`
⇒`Hyp^2=64+225 `
⇒`Hyp^2=289`
⇒`Hyp=17 cm`
So, the third side is 17 cm.
Perimeter of a triangle = a + b + c.
Therefore, required perimeter of the triangle 15 + 8 + 1740 cm
APPEARS IN
संबंधित प्रश्न
From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in the given figure. Find the area of the remaining portion of the square [Use Π = 22/7]

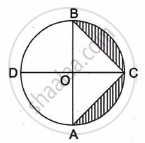
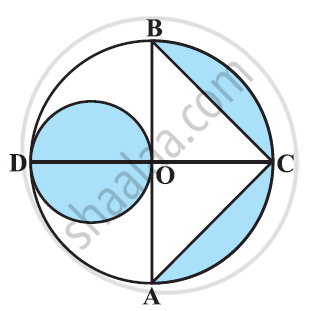
In the given figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. [Use Π = 22/7]
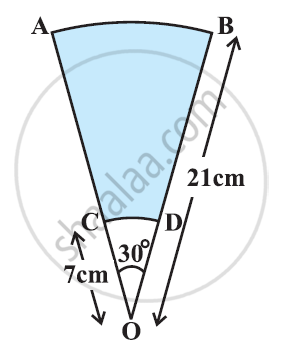
AB and CD are respectively arcs of two concentric circles of radii 21 cm and 7 cm and centre O (see the given figure). If ∠AOB = 30°, find the area of the shaded region. [Use Π = 22/7]
A park is in the form of rectangle 120m × 100m. At the centre of park there is a circular lawn. The area of park excluding lawn is 8700m2. Find the radius of circular lawn.
The area enclosed between the concentric circles is 770cm2. If the radius of outer circle 21cm. find the radius of inner circle
The perimeter of a right triangle is 40 cm and its hypotenuse measure 17 cm. Find the area of the triangle.
Each side of an equilateral triangle is 10 cm. Find (i) the area of the triangle and (ii) the height of the triangle.
Find the area of shaded region in Fig. 4, where a circle of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm. (Use π = 3.14 and\[\sqrt{3}\] =1.73)
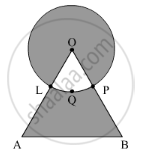
In Figure 4, ABCD is a square of side 4 cm. A quadrant of a circle of radius 1 cm is drawn at each vertex of the square and a circle of diameter 2 cm is also drawn. Find the area of the shaded region. (Use π = 3.14)
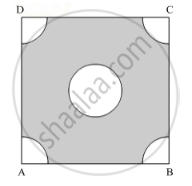
In the given figure, AB and CD are diameters of a circle with centre O perpendicular to each other. If OA = 7 cm, find the area of shaded region.