Advertisements
Advertisements
प्रश्न
The dimensions of a room are 14 m x 10 m x 6.5 m There are two doors and 4 windows in the room. Each door measures 2.5 m x 1.2 m and each window measures 1.5 m x 1 m. Find the cost of painting the four walls of the room at ₹ `35perm^2` .
उत्तर
The room has four walls to be painted
Area of these walls=`2(lxxh)+2(bxxh)`
=`(2xx14xx6.5)+(2xx10xx6.5)`
=`312m^2`
Now, Area of the two doors=`(2xx2.5xx1.2)=6m^2`
Area of the four windows=`(4xx1.5xx1)=6m^2`
The walls have to be painted; the doors and windows are not to be painted.
∴ Total area to be painted =`312-(6+6)=300m^2`
Cost for painting=`1m^2=Rs35`
Cost for painting `300m^2=300xx35=Rs10,500`
APPEARS IN
संबंधित प्रश्न
In the following figure, the square ABCD is divided into five equal parts, all having same area. The central part is circular and the lines AE, GC, BF and HD lie along the diagonals AC and BD of the square. If AB = 22 cm, find:
the perimeter of the part ABEF.

Find the area of the largest triangle that can be inscribed in a semi-circle of radius runits.
The area of the largest triangle that can be inscribed in a semi-circle of radius r, is
The perimeter of a certain sector of a circle of radius 6.5 cm in 31 cm. Find the area of the sector.
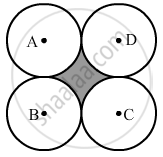
Four equal circles, each of radius 5 cm, touch each other, as shown in the figure. Find the area included between them.
On increasing the diameter of a circle by 40%, its area will be increased by
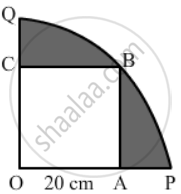
In the given figure, a square OABC has been inscribed in the quadrant OPBQ. If OA = 20 cm, then the area of the shaded region is
The following figure shows a square cardboard ABCD of side 28 cm. Four identical circles of the largest possible sizes are cut from this card as shown below.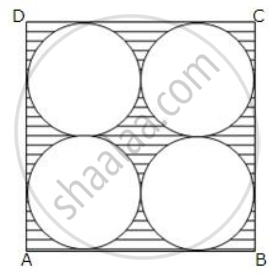
Find the area of the remaining card-board.
Find the area of the shaded portion in each of the following diagrams:
(i)

(ii)

Find the area of the dining table whose diameter is 105 cm
