Advertisements
Advertisements
प्रश्न
On increasing the diameter of a circle by 40%, its area will be increased by
विकल्प
40%
80%
96%
82%
उत्तर
96%
Let d be the original diameter.
Radius `="d"/2`
Thus, we have;
Original area `= π xx ("d"/2)`
`= (pi"a"^2)/4`
New diameter = 140% of d
`= (140/100xx"d")`
`= (7"d")/5`
Now,
New radius ` =(7"d")/5xx2`
`=(7"d")/10`
New area `= pixx((7"d")/10)^2`
`=(49π"a""^2)/10`
Increase in the area`=((49pi"a"^2)/10 - (pi"a"^2)/4)``
`=(24π"a"^2)/100`
`=(6pi"a"^2)/25 `
We have ;
Increase in the area`=((6pi"a"^2)/25xx4/(pi"a"^2)xx100)%`
= 96 %
APPEARS IN
संबंधित प्रश्न
A room is 16 m long and 13.5 m broad. Find the cost of covering its floor with 75-m-wide carpet at ₹ 60 per metre.
The area of a parallelogram is `392m^2` . If its altitude is twice the corresponding base, determined the base and the altitude.
Find the area of the shaded region in the following figure, if AC = 24 cm, BC = 10 cm and O is the centre of the circle. (Use π = 3.14)

If a chord of a circle of radius 28 cm makes an angle of 90 ° at the centre, then the area of the major segment is
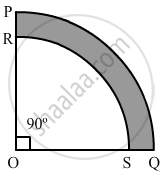
In the given figure, PQRS represents a flower bed. If OP = 21 m and OR = 14 m, find the area of the flower bed.
A circular disc of radius 6 cm is divided into three sectors with central angles 90°,120° and 150°. What part of the whole circle is the sector with central angle 150°? Also, calculate the ratio of the areas of the three sectors.
If the sum of the areas of two circles with radii R1 and R2 is equal to the area of a circle of radius R, then ______.
The length of the minute hand of a clock is 21 cm. The area swept by the minute hand in 10 minutes is
If a square in inscribed in a circle, find the ratio of the areas of the circle and the square.
A table top is semicircular in shape with diameter 2.8 m. Area of this table top is ______.
