Advertisements
Advertisements
प्रश्न
The length of the minute hand of a clock is 21 cm. The area swept by the minute hand in 10 minutes is
विकल्प
231 cm2
210 cm2
126 cm2
252 cm2
उत्तर
Angle subtends by the minute hand in 1 minute = 6∘
∴ Angle subtends by the minute hand in 10 minutes = 60∘
Now,
Area of the sector`=theta/(360°) pi"r"^2 = 60°/360° xx 22/7(21)^2 = 231 "cm"^2`
Now,
Area of the sector`=theta/360°pi"r"^2 = (60°)/360^circxx22/7(21)^2=231 "cm"^2`
Hence, the correct answer is option (a).
APPEARS IN
संबंधित प्रश्न
Each of the equal sides of an isosceles triangle measure 2 cm more than its height, and the base of the triangle measure 12 cm. Find the area of the triangle.
The cost of harvesting a square field at ₹ 900 per hectare is ₹ 8100. Find the cost of putting a fence around it at ₹ 18 per meter.
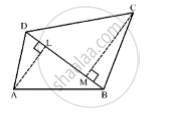
In the given figure ABCD is quadrilateral in which diagonal BD = 24 cm, AL ⊥ BD and CM ⊥ BD such that AL = 9cm and CM = 12 cm. Calculate the area of the quadrilateral.
A chord PQ of length 12 cm subtends an angle of 120° at the centre of a circle. Find the area of the minor segment cut off by the chord PQ.
A child makes a poster on a chart paper drawing a square ABCD of side 14 cm. She draws four circles with centre A, B, C and D in which she suggests different ways to save energy. The circles are drawn in such a way that each circle touches externally two of the three remaining circles (in the following figure). In the shaded region she write a message 'Save Energy'. Find the perimeter and area of the shaded region.
(Use π = 22/7)

If the sum of the areas of two circles with radii r1 and r2 is equal to the area of a circle of radius r, then \[r_1^2 + r_2^2\]
The radii of the inner and outer circumferences of a circular running track are 63 m and 70 m respectively. Find :
(i) the area of the track ;
(ii) the difference between the lengths of the two circumferences of the track.
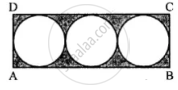
In the following figure, a rectangle ABCD enclosed three circles. If BC = 14 cm, find the area of the shaded portion (Take π = 22/7)
Find the area enclosed between two concentric circles, If their radii are 6cm and 13cm respectively.
What is the diameter of a circle whose area is equal to the sum of the areas of two circles of radii 40cm and 9cm?
