Advertisements
Advertisements
प्रश्न
The radii of the inner and outer circumferences of a circular running track are 63 m and 70 m respectively. Find :
(i) the area of the track ;
(ii) the difference between the lengths of the two circumferences of the track.
Advertisements
उत्तर
Outer radius, r1 = 70 m
Inner radius, r2 = 63 m
∴ Area of track = `pir_1^2 - pir_2^2`
= `22/7[(70)^2 - (63)^2]`
= `22/7(70 + 63)(70 - 63)`
= `22/7 xx 133 xx 7`
= 2926 m2
Length of outer edge i.e. circumference
= `2pir_1`
= `2 xx 22/7 xx 70 = 440`m
Length of inner edge = `2pir_2`
= `2 xx 22/7 xx 63 = 396` m
Difference between lengths of two circumferences = 440 – 396 = 44 m
Hence (i) 2926 m2 (ii) 44 m
APPEARS IN
संबंधित प्रश्न
A circular flower garden has an area of 314 m2. A sprinkler at the centre of the garden can cover an area that has a radius of 12 m. Will the sprinkler water the entire garden? (Take π = 3.14)
In the following figure, ABCD is a rectangle, having AB = 20 cm and BC = 14 cm. Two sectors of 180° have been cut off. Calculate:
the length of the boundary of the shaded region.
In the the following figure, PSR, RTQ and PAQ are three semicircles of diameter 10 cm, 3 cm and 7 cm respectively. Find the perimeter of shaded region.
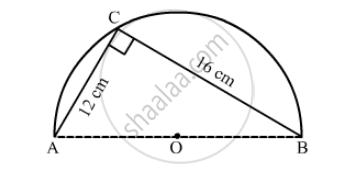
In the following figure, O is the centre of a circular arc and AOB is a straight line. Find the perimeter and the area of the shaded region correct to one decimal place. (Take π = 3.142)
If area of a circle inscribed in an equilateral triangle is 48π square units, then perimeter of the triangle is
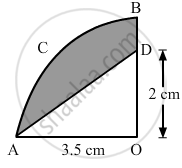
In the given figure, OABC is a square of side 7 cm. If COPB is a quadrant of a circle with centre C find the area of the shaded region.
Find the areas of both the segments of a circle of radius 42 cm with central angle 120°. [Given, sin 120° = `sqrt(3)/2` and `sqrt(3) = 1.73`.]
The length of the minute hand of a clock is 21 cm. The area swept by the minute hand in 10 minutes is ______.
The minute hand of a clock is 8 cm long. Find the area swept by the minute hand between 8.30 a.m. and 9.05 a.m.
The sum of the radii of two circles is 10.5 cm and the difference of their circumferences is 13.2 cm. Find the radii of the two circles.
