Advertisements
Advertisements
प्रश्न
The length of the tangent to a circle from a point P, which is 25 cm away from the centre is 24 cm. What is the radius of the circle?
उत्तर
Let the radius AB be r.
In the right ∆ABO,
OB2 = OA2 + AB2
252 = 242 + r2
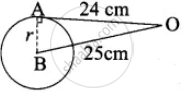
252 – 242 = r2
(25 + 24) (25 – 24) = r2
r = `sqrt(49)` = 7
Radius of the circle = 7 cm
APPEARS IN
संबंधित प्रश्न
A tangent ST to a circle touches it at B. AB is a chord such that ∠ABT = 65°. Find ∠AOB, where “O” is the centre of the circle.
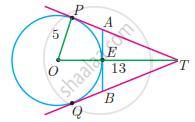
O is the centre of the circle with radius 5 cm. T is a point such that OT = 13 cm and OT intersects the circle E, if AB is the tangent to the circle at E, find the length of AB.
In two concentric circles, a chord of length 16 cm of larger circle becomes a tangent to the smaller circle whose radius is 6 cm. Find the radius of the larger circle
Two circles with centres O and O’ of radii 3 cm and 4 cm, respectively intersect at two points P and Q, such that OP and O’P are tangents to the two circles. Find the length of the common chord PQ.
Draw a tangent at any point R on the circle of radius 3.4 cm and centre at P?
Draw the two tangents from a point which is 5 cm away from the centre of a circle of diameter 6 cm. Also, measure the lengths of the tangents.
A tangent is perpendicular to the radius at the
How many tangents can be drawn to the circle from an exterior point?
CP and CQ are tangents to a circle with centre at 0. ARB is another tangent touching the circle at R. If CP = 11 cm and BC = 7 cm, then the length of BR is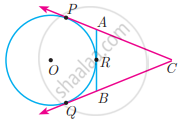
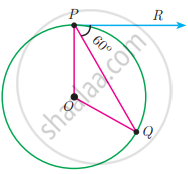
If PR is tangent to the circle at P and O is the centre of the circle, then ∠POQ is