Advertisements
Advertisements
प्रश्न
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
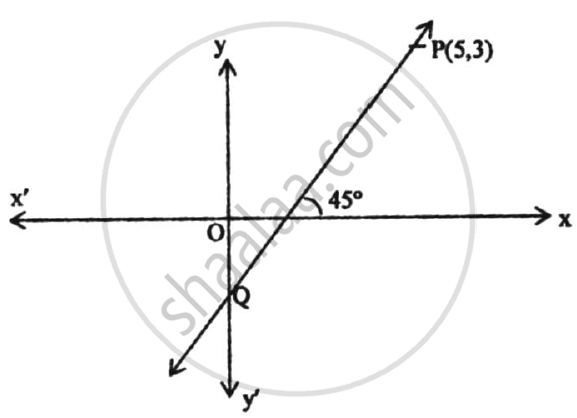
उत्तर
1) Since the line make 45o with the positive direction of x-axis,
Therefore slope (m) = tan 45° = 1
2) An equation of a line, using point-slope is
`y - y_1 = m(x - x_1)`
`=> y - 3 = 1 (x - 5)`
`=> y - 3 = x - 5`
`=> y = x - 2`
3) Since Q is a point on y-axis, its x-coordinate is zero.
Putting x = 0 in the equation of line, we get y = –2
Hence the point Q is (0, –2).
APPEARS IN
संबंधित प्रश्न
The line passing through (0, 2) and (−3, −1) is parallel to the line passing through (−1, 5) and (4, a). Find a.
Plot the points A(1, 1), B(4, 7) and C(4, 10) on a graph paper. Connect A and B and also A and C.
Which segment appears to have the steeper slope, AB or AC?
Justify your conclusion by calculating the slopes of AB and AC.
Find the slope of the lines passing through the given point.
C (5, –2) , D (7, 3)
Find the slope of the lines passing through the given point.
T (0, –3) , S (0, 4)
Find k, if R(1, –1), S (–2, k) and slope of line RS is –2.
Determine whether the given point is collinear.
A (0, 2), B (1, -0.5), C (2, -3)
Determine whether the given point is collinear.
L(1,2), M(5,3) , N(8,6)
Show that points P(1, –2), Q(5, 2), R(3, –1), S(–1, –5) are the vertices of a parallelogram.
Find the slope of a line parallel to the given line x +3y = 7
The line through A (- 2, 3) and B (4, b) is perpendicular to the line 2a – 4y = 5. Find the value of b.
