Advertisements
Advertisements
प्रश्न
The points (2, -1), (-1, 4) and (-2, 2) are midpoints of the sides ofa triangle. Find its vertices.
उत्तर
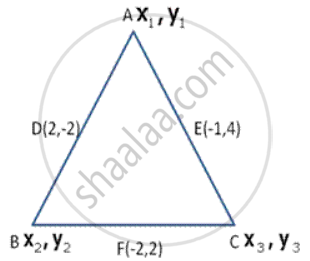
Let A(x1,y1), B(x2, y2 ) and C(x3, y3) be the coordinates of the vertices of Δ ABC.
Midpoint of AB, i.e. D
D (2 , 1) = D `(("x"_1 + "x"_2)/2 , ("y"_1 + "y"_2)/2)`
`2 = ("x"_1 + "x"_2)/2 , ("y"_1 + "y"_2)/2` = -1
X1 + X2 = 4 .....(1) Y1 + Y2 = -2 ........(2)
Similarly,
X1 + X3 = -2 .....(3) y1 + y3 = 8 . ....(4)
X2 + X3 = -4 .....(5) Y2 + Y3 = 4 .....(6)
Adding (1), (3) and (5)
2(x1 + x2 + x3) = -2
x1 + x2 + x3 = -1
4 + x3 = -1 ... (from (1))
x3 = -5
From (3)
x1 - 5 = -2
x1 = 3
From (5)
x2 = -5 = -4
x2 = 1
Adding (2),(4) and (6)
2( Y1 + Y2 + Y3)= 10
Y1 +Y2 +Y3 = 5
-2 + Y3 = 5 [from(2)]
y3 = 7
From (4)
y1 + 7 = 8
y1 = 1
From (6)
y2 + 7 = 4
y2 = -3
The coordinates of the vertices of A.ABC are (3, 1), ( 1,-3) and ( -5,7)
APPEARS IN
संबंधित प्रश्न
Given M is the mid-point of AB, find the co-ordinates of B; if A = (3, –1) and M = (–1, 3).
One end of the diameter of a circle is (–2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, –1).
Prove that the points A(–5, 4); B(–1, –2) and C(5, 2) are the vertices of an isosceles right-angled triangle. Find the co-ordinates of D so that ABCD is a square.
Find the length of the median through the vertex A of triangle ABC whose vertices are A (7, -3), B(S, 3) and C(3, -1).
The coordinates of the centroid I of triangle PQR are (2, 5). If Q = (-6, 5) and R = (7, 8). Calculate the coordinates of vertex P.
If P(–b, 9a – 2) divides the line segment joining the points A(–3, 3a + 1) and B(5, 8a) in the ratio 3: 1, find the values of a and b.
The coordinates of the point C dividing the line segment joining the points P(2, 4) and Q(5, 7) internally in the ratio 2 : 1 is
The coordinates of diameter AB of a circle are A(2, 7) and B(4, 5), then find the coordinates of the centre
Find the coordinates of midpoint of segment joining (22, 20) and (0, 16)
If A(5, 4), B(–3, –2) and C(1, –8) are the vertices of a ∆ABC. Segment AD is median. Find the length of seg AD:
