Advertisements
Advertisements
प्रश्न
The sum of three consecutive multiples of 8 is 888. Find the multiples.
उत्तर
Let the three consecutive multiples of 8 be 8x, 8(x + 1), 8(x + 2).
Sum of these numbers = 8x + 8(x + 1) + 8(x + 2) = 888
8(x + x + 1 + x + 2) = 888
8(3x + 3) = 888
On dividing both sides by 8, we obtain
`(8(3x + 3))/8 = 888/8`
3x + 3 = 111
On transposing 3 to R.H.S, we obtain
3x = 111 − 3
3x = 108
On dividing both sides by 3, we obtain
`3x/3 = 108/3`
x = 36
First multiple = 8x = 8 × 36 = 288
Second multiple = 8(x + 1) = 8 × (36 + 1) = 8 × 37 = 296
Third multiple = 8(x + 2) = 8 × (36 + 2) = 8 × 38 = 304
Hence, the required numbers are 288, 296, and 304
APPEARS IN
संबंधित प्रश्न
The ages of Rahul and Haroon are in the ratio 5:7. Four years later the sum of their ages will be 56 years. What are their present ages?
What should be added to twice the rational number `(-7)/3 "to get" 3/7`?
Three consecutive numbers whose sum is 12 are ______, ______ and ______.
3 years ago, the age of a boy was y years. His age 2 years ago was (y – 2) years.
The sum of four consecutive integers is 266. What are the integers?
A man was engaged as typist for the month of February in 2009. He was paid Rs. 500 per day but Rs. 100 per day were deducted for the days he remained absent. He received Rs. 9,100 as salary for the month. For how many days did he work?
There are 40 passengers in a bus, some with Rs. 3 tickets and remaining with Rs. 10 tickets. The total collection from these passengers is Rs. 295. Find how many passengers have tickets worth Rs. 3?
A steamer goes downstream and covers the distance between two ports in 5 hours while it covers the same distance upstream in 6 hours. If the speed of the stream is 1 km/hr, find the speed of the steamer in still water.
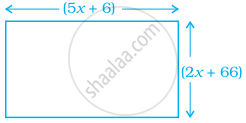
For what value of x is the perimeter of shape 186 cm?