Advertisements
Advertisements
प्रश्न
Trapezium given below; find its area.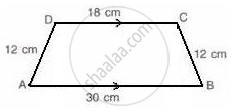
Advertisements
उत्तर
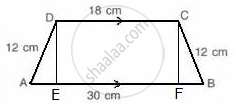
In the given figure, we can observe that the non-parallel sides are equal and hence it is an isosceles trapezium.
Therefore, let us draw DE and CF perpendiculars to AB.
Thus, the area of the parallelogram is given by
AB = AE + EF + FB and CD = EF = 18 cm, we have
30 = AE + 18 + FB
⇒ 30 = AE + 18 + AE
⇒ 2AE + 18 = 30
⇒ 2AE = 30 - 18
⇒ 2AE = 12
⇒ AE = 6 cm
Now, consider the right angled triangle ADE.
AD2 = AE2 + DE2
⇒ 122 = 62 + DE2
⇒ 144 = 36 + DE2
⇒ DE2 = 144 - 36
⇒ DE2 = 108
⇒ DE = `sqrt( 36 xx 3 )`
⇒ DE = `6sqrt3`
Area( `square"ABCD" ) = "Area"( Δ"ADE" ) + "Area"( square "DEFC") + "Area"( Δ"CFB" )`
⇒ Area( `square"ABCD" ) = 1/2 xx 6 xx 6sqrt3 + 18 xx 6sqrt3 + 1/2 xx 6 xx 6sqrt3`
⇒ Area( `square"ABCD" ) = 6 xx 6sqrt3 + 18 xx 6sqrt3`
⇒ Area( `square"ABCD") = 144sqrt3`= 249.41 cm2
APPEARS IN
संबंधित प्रश्न
A wire when bent in the form of a square encloses an area = 576 cm2. Find the largest area enclosed by the same wire when bent to form;
(i) an equilateral triangle.
(ii) A rectangle whose adjacent sides differ by 4 cm.
The perimeter of a rectangular board is 70 cm. Taking its length as x cm, find its width in terms of x.
If the area of the rectangular board is 300 cm2; find its dimensions.
Trapezium given below; find its area.
A wire when bent in the form of a square encloses an area of 484 m2. Find the largest area enclosed by the same wire when bent to from:
- An equilateral triangle.
- A rectangle of length 16 m.
The figure given below shows the cross-section of a concrete structure. Calculate the area of cross-section if AB = 1.8 cm, CD = 0.6 m, DE = 0.8 m, EF = 0.3 m and AF = 1.2 m.
The width of a rectangular room is `4/7`of its length, x, and its perimeter is y. Write an equation connecting x and y. Find the length of the room when the perimeter is 4400 cm.
The perimeter of a rhombus is 46 cm. If the height of the rhombus is 8 cm; find its area.
The length of a rectangular verandah is 3 m more than its breadth. The numerical value of its area is equal to the numerical value of its perimeter.
(i) Taking x as the breadth of the verandah, write an equation in x that represents the above statement
(ii) Solve the equation obtained in (i) above and hence find the dimensions of the verandah.
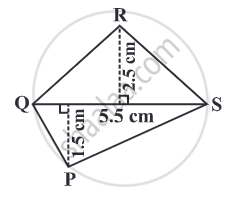
Find the area of quadrilateral BCEG
PQRS is a rectangle formed by joining the points P(– 1, – 1), Q(– 1, 4), R(5, 4) and S(5, – 1). A, B, C and D are the mid-points of PQ, QR, RS and SP respectively. Is the quadrilateral ABCD a square, a rectangle or a rhombus? Justify your answer.
