Advertisements
Advertisements
प्रश्न
Two circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T; show that the points P, B, Q and T are concyclic.
उत्तर
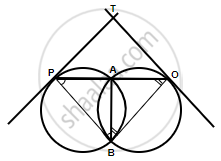
Join AB, PB and BQ
TP is the tangent and PA is a chord
∴ ∠TPA = ∠ABP ...(i) (Angles in alternate segment)
Similarly,
∠TQA = ∠ABQ ...(ii)
Adding (i) and (ii)
∠TPA + ∠TQA = ∠ABP + ∠ABQ
But, ΔPTQ,
∠TPA + ∠TQA + ∠PTQ = 180°
`=>` ∠PBQ = 180° – ∠PTQ
`=>` ∠PBQ + ∠PTQ = 180°
But they are the opposite angles of the quadrilateral
Therefore, PBQT are cyclic.
Hence, P, B, Q and T are concyclic.
APPEARS IN
संबंधित प्रश्न
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

(ii) angles APB = 90°
Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that ∠CPA = ∠DPB.
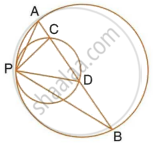
Two circles intersect each other at points A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.

Radius of a sector of a circle is 21 cm. If length of arc of that sector is 55 cm, find the area of the sector.
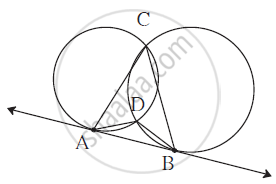
Two circles intersect each other at points C and D. Their common tangent AB touches the circles at point A and B. Prove that :
∠ ADB + ∠ ACB = 180°
In which qudrant does point A(-3, 2) lie?
On which axis does point B(12, 0) lie?
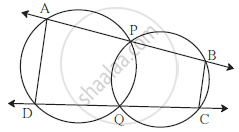
Two circles intersect each other at points P and Q. Secants drawn through P and Q intersect the circles at points A,B and D,C. Prove that : ∠ADC + ∠BCD = 180°
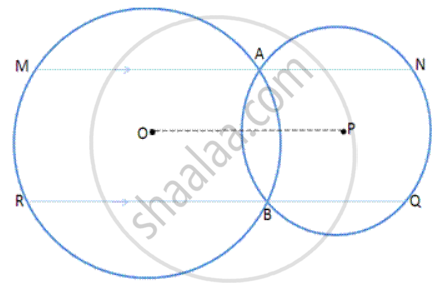
Two circles with centres O and P intersect each other at A and B as shown in following fig. Two straight lines MAN and RBQ are drawn parallel to OP.
Prove that (i) MN = 20 P (ii) MN= RQ.
Two circles of radii 5cm and 3cm with centres O and P touch each other internally. If the perpendicular bisector of the line segment OP meets the circumference of the larger circle at A and B, find the length of AB.
Two circles with centres O and O' touch each other at point L. Prove that, a tangent through L bisects the common tangent AB of the two circles at point M.

Given: AB is a common tangent of the two circles that touch each other at point L. ML is a tangent through point L.
To prove: M is a mid-point of the tangent AB or MA = MB.
Proof: From the figure,
M is an external point that draws two tangents, MA and ML to the circle with the centre O.
So, `square` = `square` ......(i)
Similarly, M draws two tangents ML and MB to the circle with the centre O'.
So, `square` = `square` ......(ii)
From the equations (i) and (ii),
`square` = `square`
Hence, the tangent at the point L, bisects the common tangent, AB of the two circles at point M.
