Advertisements
Advertisements
प्रश्न
Two straight lines are cut by a transversal. Are the corresponding angles always
उत्तर
If a transversal cuts two straight lines, their the corresponding angles are not equal unless the lines are not parallel. One in case of parallel lines, the corresponding angles are equal.
APPEARS IN
संबंधित प्रश्न
In your note-book copy the following angle using ruler and a pair compass only.
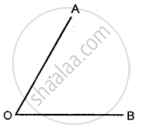
Construct the 60° angle, using ruler and a pair of compass only.
Construct the 135° angle, using ruler and a pair of compass only.
Draw a line segment PQ = 8cm. Construct the perpendicular bisector of the line segment PQ. Let the perpendicular bisector drawn meet PQ at point R. Measure the lengths of PR and QR. Is PR = QR?
Draw a line segment AB = 7cm. Mark a point Pon AB such that AP=3 cm. Draw perpendicular on to AB at point P.
Draw ∠ABC = 120°. Bisect the angle using ruler and compasses. Measure each angle so obtained and check whether or not the new angles obtained on bisecting ∠ABC are equal.
Two straight lines are cut by a transversal so that the co-interior angles are supplementary. Are the straight lines parallel?
Two straight lines are cut by a transversal so that the co-interior angles are equal. What must be the measure of each interior angle to make the straight lines parallel to each other?
Draw a line segment AB = 6 cm. Without using set squares, draw angle OAB = 60° and angle OBA = 90°. Measure angle AOB and write this measurement.
Without using set squares, construct angle ABC = 60° in which AB = BC = 5 cm. Join A and C and measure the length of AC.
