Advertisements
Advertisements
प्रश्न
In your note-book copy the following angle using ruler and a pair compass only.
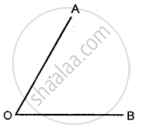
उत्तर
Steps of Construction:
1. At point Q, draw line QR = OB.

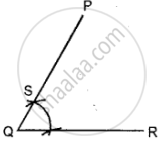
2. With O as a centre, draw an arc of any suitable radius, to cut the arms of the angle at C and D.
3. With Q as a centre, draw the arc of the same size as drawn for C and D. Let this arc cuts line QR at point T.
4. In your compasses, take the distance equal to distance between C and D; and then with T as a centre, draw an arc which cuts the earlier arc at S.
5. Join QS and produce up to a suitable point P. ∠PQR so obtained, is the angle equal to the given ∠AOB.
APPEARS IN
संबंधित प्रश्न
The adjoining figure shows two straight lines AB and CD intersecting at point P. If ∠BPC = 4x – 5° and ∠APD = 3x + 15°; find:

(i) the value of x.
(ii) ∠APD
(iii) ∠BPD
(iv) ∠BPC
In your note-book copy the following angle using ruler and a pair compass only.
Construct the 120° angle, using ruler and a pair of compass only.
Construct the 15° angle, using ruler and a pair of compass only.
Draw line AB = 6 cm. Construct angle ABC = 60°. Then draw the bisector of angle ABC.
Draw a line segment PQ = 8cm. Construct the perpendicular bisector of the line segment PQ. Let the perpendicular bisector drawn meet PQ at point R. Measure the lengths of PR and QR. Is PR = QR?
Draw ∠ABC = 120°. Bisect the angle using ruler and compasses. Measure each angle so obtained and check whether or not the new angles obtained on bisecting ∠ABC are equal.
Two straight lines are cut by a transversal. Are the corresponding angles always
Draw a line AB = 9 cm. Mark a point P in AB such that AP=5 cm. Through P draw (using set-square) perpendicular PQ = 3 cm. Measure BQ.
Without using set squares, construct angle ABC = 60° in which AB = BC = 5 cm. Join A and C and measure the length of AC.
