Advertisements
Advertisements
प्रश्न
x ∈ {real numbers} and –1 < 3 – 2x ≤ 7, evaluate x and represent it on a number line.
उत्तर
–1 < 3 – 2x ≤ 7
–1 < 3 – 2x and 3 – 2x ≤ 7
2x < 4 and – 2x ≤ 4
x < 2 and x ≥ – 2
Solution set = {–2 ≤ x < 2, x ∈ R}
Thus, the solution can be represented on a number line as:
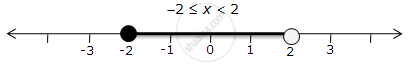
APPEARS IN
संबंधित प्रश्न
If the replacement set is the set of whole numbers, solve:
3x – 1 > 8
If the replacement set is the set of real numbers, solve:
`5 + x/4 > x/5 + 9`
Given x ∈ {whole numbers}, find the solution set of:
–1 ≤ 3 + 4x < 23
For graph given alongside, write an inequation taking x as the variable:
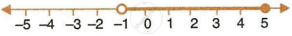
Find the range of values of x which satisfies
`-2 2/3 <= x + 1/3 < 3 1/3, x in R`
Graph these values of x on the number line.
Solve for x in the following in-equation, if the replacement set is R;
2x - 3 < 7
Solve for x in the following in-equation, if the replacement set is R;
3x + 2 ≤ 11
Solve for x in the following in-equation, if the replacement set is R;
7x + 11 > 16 - 3x
Solve for x in the following in-equation, if the replacement set is R;
9 - 4x ≤ 15 - 7x
Find the greatest integer which is such that if 7 is added to its double, the resulting number becomes greater than three times the integer.
