Advertisements
Advertisements
प्रश्न
A car travels the first 30 km with a uniform speed of 60 km h-1 and the next 30 km with a uniform speed of 40 km h-1. Calculate :
The total time of journey,
The average speed of the car.
उत्तर
For the first 30 km travelled, speed = 60 km/h.
Thus time taken (t1) = Distance / speed
= (30/60) h-1
= 0.5 h-1 or 30 min.
For the next 30 km travelled, speed = 40 km/h
Thus time taken (t2) = Distance/speed
= (30/40) h-1
= 0.75 h-1 or 45 min.
(i) Total time = (30 + 45) min
= 75 min or 1.25 h.
(ii) Average speed of the car = Total distance travelled/total time taken = `(60 "km")/(1.25 "hr")` = 48 km h-1
APPEARS IN
संबंधित प्रश्न
An aircraft travelling at 600 km/h accelerates steadily at 10 km/h per second. Taking the speed of sound as 1100 km/h at the aircraft’s altitude, how long will it take to reach the ‘sound barrier’ ?
A car is moving along a straight road at a steady speed. It travels 150 m in 5 seconds:
How far does it travel in 6 seconds ?
A train starting from rest moves with a uniform acceleration of 0.2 m/s2 for 5 minutes. Calculate the speed acquired and the distance travelled in this time.
Which of the following could not be a unit of speed?
The velocity-time graph of a moving body is given below in Figure
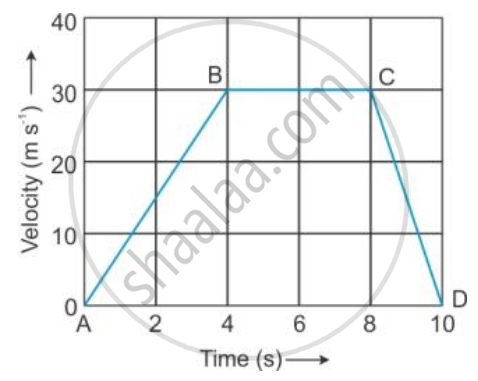
The acceleration in parts AB, BC and CD.
A train travels with a speed of 60 km h-1 from station A to station B and then comes back with a speed 80 km h-1 from station B to station A. Find -
- The average speed
- The average velocity of the train.
Define variable velocity and give one example.
Define average velocity and give one example.
What is the relation between distance and time when the body is moving with variable velocity?
Write down the type of motion of a body along with the A – O – B of the following distance – time graph.