Advertisements
Advertisements
प्रश्न
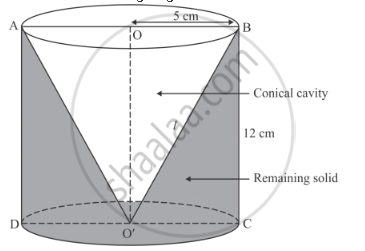
A conical hole is drilled in a circular cylinder of height 12 cm and base radius 5 cm. The height and the base radius of the cone are also the same. Find the whole surface and volume of the remaining cylinder.
उत्तर
Given that:
r = 5 cm
h = 12 cm
We have the following diagram
Slant height of cone is given by
`l=sqrt(r^2+h^2)`
`=sqrt(5^2+12^2`
= 13 cm
The total surface area of the remaining part is given by
`S=2pirh+pir^2+pirl`
`2xxpixx5xx12+pixx5^2+pixx5xx13`
= 120π+25π+65π
=210π cm2
The volume of the remaining part is given by
`V=pir^2h-1/3pir^2h`
`=2/3pir^2h`
`=2/3xxpixx5^2xx12`
= 200π cm3
Hence, S =210π cm2. V = 200π cm3
APPEARS IN
संबंधित प्रश्न
Volume and surface area of a solid hemisphere are numerically equal. What is the diameter of hemisphere?
Water is flowing through a cylindrical pipe, of internal diameter 2 cm, into a cylindrical tank of base radius 40 cm, at the rate of 0.4 m/s. Determine the rise in level of water in the tank in half an hour ?
A metal parallelopiped of measures 16 cm x 11 cm x 10 cm was melted to make coins. How many coins were made if the thickness and diameter of each coin were 2 mm and 2 cm respectively?
A solid metallic sphere of radius 5.6 cm is melted and solid cones each of radius 2.8 cm and height 3.2 cm are made. Find the number of such cones formed.
A hemispherical bowl of internal radius 9 cm is full of liquid . The liquid is to be filled into cylindrical shaped bottles each of radius 1.5 cm and height 4 cm . How many bottles are needed to empty the bowl ?
A factory manufactures 120,000 pencils daily . The pencil are cylindrical in shape each of length 25 cm and circumference of base as 1.5 cm . Determine the cost of colouring the curved surfaces of the pencils manufactured in one day at ₹0.05 per dm2.
The height of a solid cylinder is 15 cm and the diameter of its base is 7 cm. Two equal conical holes each of radius 3 cm and height 4 cm are cut off. Find the volume of the remaining solid.
A golf ball has diameter equal to 4.2 cm. Its surface has 200 dimples each of radius 2 mm. Calculate the total surface area which is exposed to the surroundings assuming that the dimples are hemispherical.
A toy is in the form of a cone mounted on a hemisphere of common base radius 7 cm. The total height of the toy is 31 cm. Find the total surface area of the toy.
Ratio of area of a circle to the area of a square whose side equals radius of circle is 1 : π.
