Advertisements
Advertisements
प्रश्न
A family is using Liquefied petroleum gas (LPG) of weight 14.2 kg for consumption. (Full weight 29.5kg includes the empty cylinders tare weight of 15.3kg.). If it is used with constant rate then it lasts for 24 days. Then the new cylinder is replaced. Draw the graph for first 96days
उत्तर
The relation connecting the quantity of gas to the number of days of consumption is
y = `- 71/120 x + 14.2`
Let f(x) = `- 71/120 x + 14.2`
Here f(x) is a periodic function of period 24
∴ f(x + 24) = f(x)
When x = 0
f(0) = `- 71/120 xx 0 + 14.2`
⇒ y = 14.2
The corresponding point is (0, 14.2)
When x = 24
f(24) = `- 71/120 xx 0 + 14.2`
⇒ y = 14.2
⇒ f(24) = `- 71/5 + 14.2`
= – 14.2 + 14.2 = 0
⇒ y = 0
Corresponding point is (24 , 0)
When x = 48
f(48) = f(24 + 24 + 0)
= f(24 + 0)
= f(0) = 0
Corresponding point is (48, 0)
When x = 72
f(72) = f(24 + 24 + 24 + 0)
= f(24 + 24 + 0)
= f(24 + 0)
= f(0) = 0
Corresponding point is (72, 0)
The required graph is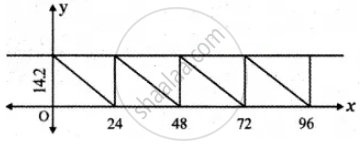
APPEARS IN
संबंधित प्रश्न
Find the slope of the following line which passes through the points:
A(2, −1), B(4, 3)
Find the slope of the following line which passes through the points:
E(2, 3), F(2, −1)
Find the slope of the following line which passes through the points:
G(7, 1), H(−3, 1)
Find the slope of the line whose inclination is 30°
Without using Pythagoras theorem show that points A(4, 4), B(3, 5) and C(−1, −1) are the vertices of a right angled triangle.
A line passes through points A(x1, y1) and B(h, k). If the slope of the line is m then show that k − y1 = m(h − x1)
Select the correct option from the given alternatives:
If A is (5, −3) and B is a point on the x-axis such that the slope of line AB is −2 then B ≡
Select the correct option from the given alternatives:
The angle between the line `sqrt(3)x - y - 2` = 0 and `x - sqrt(3)y + 1` = 0 is
Answer the following question:
Find the equation of the line containing the point T(7, 3) and having inclination 90°.
Find the equation of the lines passing through the point (1,1) and (– 2, 3)
Find the equation of the lines passing through the point (1, 1) and the perpendicular from the origin makes an angle 60° with x-axis
If p is length of perpendicular from origin to the line whose intercepts on the axes are a and b, then show that `1/("p"^3) = 1/("a"^2) + 1/("b"^2)`
The normal boiling point of water is 100°C or 212°F and the freezing point of water is 0°C or 32°F. Find the value of F for 38°C
Show that the points (1, 3), (2, 1) and `(1/2, 4)` are collinear, by using any other method
A 150 m long train is moving with constant velocity of 12.5 m/s. Find time taken to cross the bridge of length 850 m
In a shopping mall there is a hall of cuboid shape with dimension 800 × 800 × 720 units, which needs to be added the facility of an escalator in the path as shown by the dotted line in the figure. Find the minimum total length of the escalator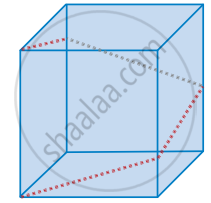
Choose the correct alternative:
The y-intercept of the straight line passing through (1, 3) and perpendicular to 2x − 3y + 1 = 0 is
If one of the lines given by kx2 + 2xy – 3y2 = 0 is perpendicular to the line 3x + 5y+ 1 = 0, then the value of k is ______.
