Advertisements
Advertisements
प्रश्न
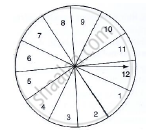
A game of chance consists of spinning an arrow which is equally likely to come to rest
pointing to one of the number, 1, 2, 3, ..., 12 as shown in Fig. below. What is the
probability that it will point to a number which is multiple of 3?
उत्तर
Total no. of possible outcomes = 12 {1, 2, 3,…., 12}
Let E ⟶ event of pointing at a no. multiple of 3
No. favourable outcomes = 4 {3, 6, 9, 12}
P(E) =`"No.of favorable outcomes"/"Total no.of possible outcomes"`
P(E) =4/12 =1/3
APPEARS IN
संबंधित प्रश्न
A lot consists of 144 ball pens of which 20 are defective and the others are good. Nuri will buy a pen if it is good, but will not buy if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that she will buy it?
A die is numbered in such a way that its faces show the number 1, 2, 2, 3, 3, 6. It is thrown two times and the total score in two throws is noted. Complete the following table which gives a few values of the total score on the two throws:
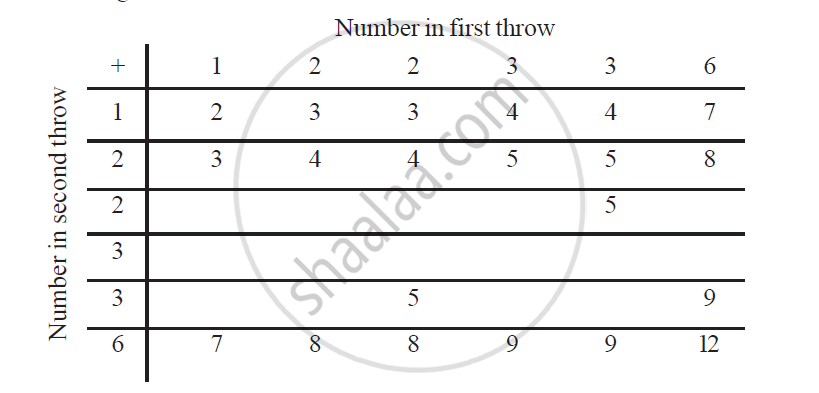
What is the probability that the total score is
(i) even? (ii) 6? (iii) at least 6?
In a lottery there are 10 prizes and 25 blanks. What is the probability of getting a prize?
A bag contains twenty Rs 5 coins, fifty Rs 2 coins and thirty Re 1 coins. If it is equally likely that one of the coins will fall down when the bag is turned upside down, what is the probability that the coin will be a Re 1 coin?
From a deck of 52 cards, all the face cards are removed and then the remaining cards are shuffled. Now one card is drawn from the remaining deck. Find the probability that the card drawn is 8 of red color.
A die is rolled twice. Find the probability that 5 will come up exactly one time.
Two dice are rolled once. Find the probability of getting such numbers on two dice whose product is a perfect square.
The probability of getting a bad egg in a lot of 400 is 0.035. The number of bad eggs in the lot is ______.
A lot consists of 48 mobile phones of which 42 are good, 3 have only minor defects and 3 have major defects. Varnika will buy a phone if it is good but the trader will only buy a mobile if it has no major defect. One phone is selected at random from the lot. What is the probability that it is acceptable to Varnika?
Complete the following table.
| Sr. No. | Random experiment | Sample space | Number of sample points in S |
| 1. | One coin is tossed. | S = {H, T} | n(S) = `square` |
| 2. | Two coins are tossed. | S = `square` | n(S) = `square` |
| 3. | Three coins are tossed. | S = {`square`, THH, HTT, THT, TTH, TTT} | n (S) = 8 |
| 4. | A die is thrown. | S = `square` | n(S) = `square` |
