Advertisements
Advertisements
प्रश्न
A hollow sphere of external and internal diameters 8 cm and 4 cm, respectively is melted into a solid cone of base diameter 8 cm. Find the height of the cone.
उत्तर
We have,
External radius of the hollow sphere, `"R"1 = 8/2 =4 "cm"`
Internal radius of the hollow sphere, `"R"2 = 4/2 = 2 "cm" and`
Let the height of the cone, `r = 8/2 = 4 "cm"`
Let the height of the cone be h.
Now, Volume of the cone = Volume of the hollow sphere
`=> 1/3pi"r"^2"h" = 4/3pi"R"_1^3 - 4/3pi"R"_2^3`
`=> 1/3pi"r"^2"h" = 4/3pi("R"_1^3 - "R"_2^3)`
`=> "h" = 4/"r"^2 ("R"_1^3 - "R"_2^3)`
`=> "h" = 4/(4xx4) (4^3 - 2^3)`
`=>"h" = 1/4(64-8)`
`=> "h" = 1/4xx56`
∴ h = 14 cm
So, the height of the cone is 14 cm.
APPEARS IN
संबंधित प्रश्न
A cylindrical vessel having diameter equal to its height is full of water which is poured into two identical cylindrical vessels with diameter 42cm and height 21cm which are filled completely. Find the diameter of cylindrical vessel?
A well of diameter 2m is dug14m deep. The earth taken out of it is spread evenly all around it to form an embankment of height 40cm. Find width of the embankment?
A copper rod of diameter 1cm and length 8cm is drawn into a wire of length 18m of uniform thickness. Find thickness of wire?
A well with inner radius 4m is dug 14m deep earth taken out of it has been spread evenly all around a width of 3m it to form an embankment. Find the height of the embankment?
A metallic right circular cone 20cm high and whose vertical angle is 90° is cut into two parts at the middle point of its axis by a plane parallel to base. If frustum so obtained bee
drawn into a wire of diameter
(1/16) cm find length of the wire?
A solid piece of iron in the form a cuboid of dimensions (49 cm × 33 cm × 24 cm) is moulded into a solid sphere. The radius of the sphere is ______.
A metallic cylinder of radius 8 cm and height 2 cm is melted and converted into a right circular cone of height 6 cm. The radius of the base of this cone is
The figure shows a running track surrounding a grassed enclosure PQRSTU. The enclosure consists of a rectangle PQST with a semicircular region at each end, PQ = 200 m; PT = 70 meters.
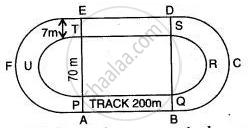
(i) Calculate the area of the grassed enclosure in m2.
(ii) Given that the track is of constant width 7 m, calculate the outer perimeter ABCDEF of the track. (Take π = `22/7`)
An iron pillar has some part in the form of a right circular cylinder and remaining in the form of a right circular cone. The radius of the base of each of cone and cylinder is 8 cm. The cylindrical part is 240 cm high and the conical part is 36 cm high. Find the weight of the pillar if one cubic cm of iron weight is 7.8 grams.
How many cubic centimetres of iron is required to construct an open box whose external dimensions are 36 cm, 25 cm and 16.5 cm provided the thickness of the iron is 1.5 cm. If one cubic cm of iron weighs 7.5 g, find the weight of the box.
